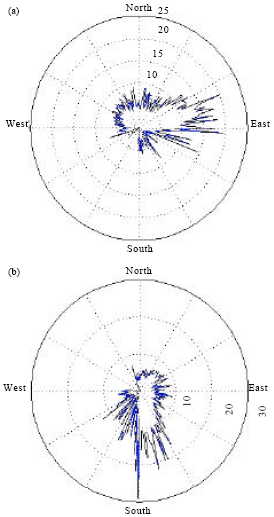
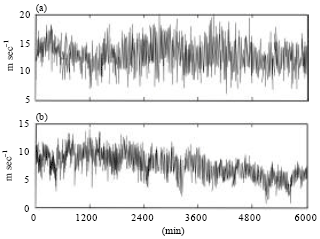
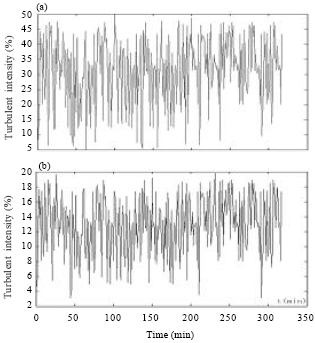
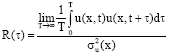Research Article
Wind Characteristics Around a Long-span Bridge in Mountainous Terrain
Chongqing Technology and Business Institute, 400052, Chongqing, China
Liangliang Zhang
College of Civil Engineering, Chongqing University, 400045, Chongqing, China
Jingyun Hang
College of Civil Engineering, Chongqing University, 400045, Chongqing, China
Haiyang Zhang
College of Civil Engineering, Chongqing University, 400045, Chongqing, China