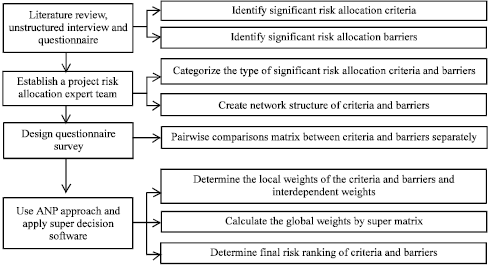
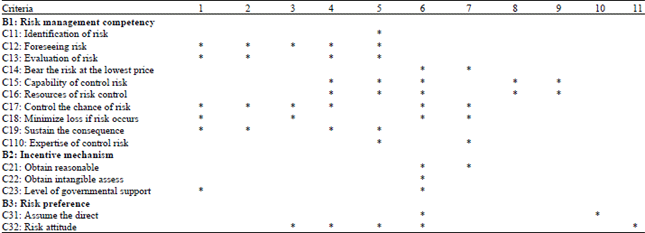
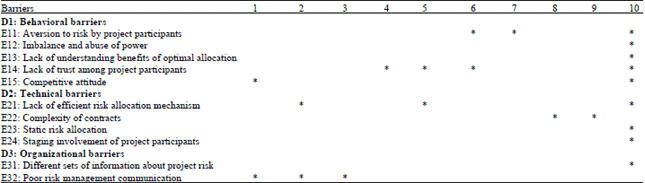
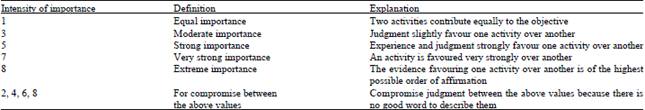
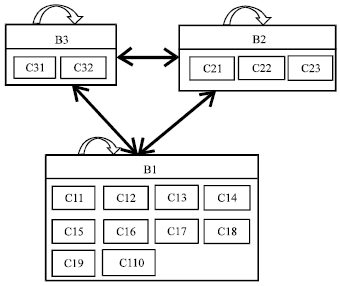
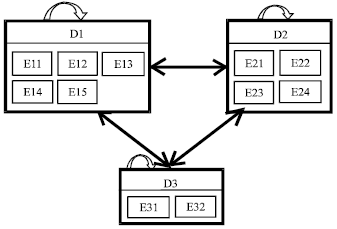
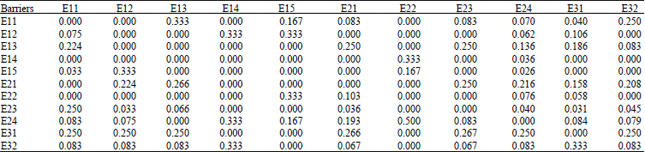
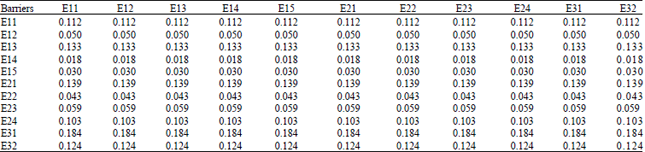
Research Article
Identification and Evaluation of Risk Allocation Criteria and Barriers: A Malaysian Public Private Partnership Project Case Study
Faculty of Civil Engineering, Universiti Teknologi Malaysia, Skudai, Johor, 81310, Malaysia
Farahbod Mohammadi
Faculty of Civil Engineering, Universiti Teknologi Malaysia, Skudai, Johor, 81310, Malaysia
Nordin Yahaya
Faculty of Civil Engineering, Universiti Teknologi Malaysia, Skudai, Johor, 81310, Malaysia
Hadi Sarvari
Faculty of Civil Engineering, Universiti Teknologi Malaysia, Skudai, Johor, 81310, Malaysia
Norhazilan Md Noor
Faculty of Civil Engineering, Universiti Teknologi Malaysia, Skudai, Johor, 81310, Malaysia