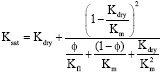Research Article
Rock Physics Modeling and Fluid Substitution Studies in Sandstone Reservoir
Faculty of Geoscience and Petroleum Engineering, Universiti Teknologi PETRONAS, Seri Iskandar, Tronoh, 31750, Perak Darul Ridzuan, Malaysia
H. Khairy
Faculty of Geoscience and Petroleum Engineering, Universiti Teknologi PETRONAS, Seri Iskandar, Tronoh, 31750, Perak Darul Ridzuan, Malaysia
A.L. Abdeldayem
Faculty of Geoscience and Petroleum Engineering, Universiti Teknologi PETRONAS, Seri Iskandar, Tronoh, 31750, Perak Darul Ridzuan, Malaysia
W. Ismail
Faculty of Geoscience and Petroleum Engineering, Universiti Teknologi PETRONAS, Seri Iskandar, Tronoh, 31750, Perak Darul Ridzuan, Malaysia