ABSTRACT
The aim of this study was to detect and identify factors in vehicle accidents on horizontal curves in rural roads (two-lane highways). Several factors appear to act upon the safety performance of horizontal curves. These factors include traffic volume and mix, geometric features of the curves, cross section, roadside hazards, stopping sight distance, vertical alignment superimposed on horizontal alignment, distance between curves and also between curves and the nearest intersections or bridges, pavement friction and traffic control devices. This study found that the most important independent effective variables in horizontal curves’ crashes based analysis was carried out which means significant are: degree horizontal curve (Dc), total length segment of horizontal curve (Lct), superelevation horizontal curve (Ec), length spiral curve (Lsp), shoulder width (Sw) and offset variable average daily traffic (ADT). The yielded equal CR.No = (ADT) (365) (L) (10-6) EXP (-10.5606250 +.108732Dc+0.000840Lct+ 0.096255Ec-0.584166Lsp-0.196970Sw) responses. Horizontal curves have higher crash rates than straight sections of similar length and traffic composition; this difference becomes apparent at radii less than 1000 m. The increase in crash rates becomes particularly significant at radii below 200 m.
PDF Abstract XML References Citation
How to cite this article
DOI: 10.3923/jas.2010.2814.2822
URL: https://scialert.net/abstract/?doi=jas.2010.2814.2822
INTRODUCTION
The design of the horizontal alignment, which consists of level tangents connected by circular curves, is influenced by design speed and superelevation of the curve itself. Crash rate for horizontal curves are higher than for tangent sections, with rates ranging between 1.5 and 4 times greater than on straight sections. Several factors appear to influence the safety performance of horizontal curves, including: (1) traffic volume and mix, (2) geometric features of the curves, (3) cross section, (4) roadside hazards, (5) stopping sight distance, (6) vertical alignment superimposed on horizontal alignment, (7) distance between curves and also between curves and the nearest intersections or bridges, (8) pavement friction and finally (9) traffic control devices. The improvement of horizontal curve design involves three steps. First, problem site must be identified based on its crash history and roadway conditions. Second, improvement should assess and implemented. Third, before and after any construction attempts, studies of crash performance should be conducted to assess the effectiveness of the changes (Garber and Hoel, 2009).
MATERIALS AND METHODS
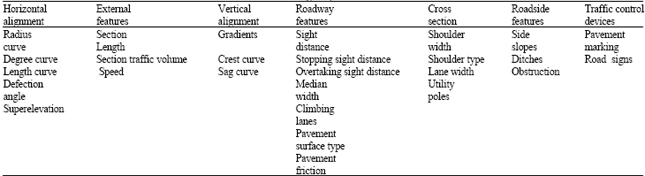
External (sectional) factors in horizontal alignment: Sectional factors include section length, section traffic volume and speed, transition curves, gradients, cross-section elements (lane width, shoulder width, median width, climbing lanes pavement friction, pavement surface type, shoulder type), roadway features (stopping sight distance, overtaking sight distance) and roadside features (side slopes, ditches, obstruction, utility poles).
Section length: Sectional factors include section length, section traffic volume and speed. A section of rural two-lane road may stretch from one county line to another and may need breaking up for the purpose of analysis. Decreasing the length of the sections has the attractive merit of ensuring (to some extent) uniform geometric features, but the smaller a segment gets, the more sparse the crash data and consequently, the less reliable the analysis will be. Furthermore, the smaller a segment becomes, the more difficult it is to assign a crash (in the current crash database) to that segment (Labi and CATS, 2006).
Section traffic volume: The traffic volume for each road section is the Average Annual Daily Traffic (AADT) on the section for a few year periods. Where the section consists of segments with different AADTs, the weighted average of the AADT over the entire section is estimated and used for the analysis. Section AADT is a very influential variable on crash frequencies. All other factors being equal, higher AADT will result in higher number of crashes, at least up to the point where capacity is reached. However, inclusion of AADT in crash modeling must be done with a great deal of circumspection because it is typically correlated with a number of explanatory variables such as lane width, shoulder width, shoulder type and pavement friction. In other words, higher volume roads are likely to have superior geometric characteristics, even within a given functional class. Such correlations were evident in the preliminary investigations for the present study.
Speed: Speed is one of the major parameters in geometric design and safety is synonymous with crash studies as mentioned by Finch et al. (1994). A recent study concluded that a reduction of 1.6 km h-1 (1 min h-1) in the average speed reduces the incidence of injuries by about 5%. Reducing rural speed limits from 100 to 90 km h-1 has been predicted to reduce casualties by about 11% (Yager and Van Aerde, 1983). It is interesting to note that the relationship between the design speed and speed limit is not referred to in the geometric design standards of many countries (O’Cinneide and McAuliffe, 1993). However, the design speed can be determined from the road standards, either using the following equation:
| (1) |
where, Vdesign design speed (km h-1), R curve radius in meters, e superelevation or crossfall in percent in meter and f side friction factor, typically 0.15 (for 120 km h-1) to 0.33 (for 30 km h-1) or estimated from the tables.
Lane width: Most studies were limited to two-lane rural roads and showed that accident rates decreased with increase in width (Hughes, 1995). However, the result of Hearne (1976) suggested that there was a marginal increase in accident occurrence with an increase in carriageway width. Hedman (1990) noted that some results indicated a rather steep decrease in accident with increased width of carriageway from 4 to 7 m, but that little additional benefit is gained by widening the carriageway beyond 7 m. This is supported by the Transportation Research Board (1978) conclusion that there is little difference between the crash rate for a 3.35 and a 3.65 m lane width. However, studies on low volume rural roads indicate that crashes continue to reduce for width greater than 3.65 m, though at a lower rate (Hughes, 1995). Yager and Van Aerde (1983) found that the passage of a vehicle requires a minimum lane width and that any additional width beyond this minimum allows one to drive faster and with a greater measure and perception of safety. For lane width from 3.3 to 3.8 m, they reported that the operation speed is decreased by approximately 5.7 km h-1 for each 1.0 m reduction of the road’s width.
Transportation Research Board (1978) suggests that widening lanes from 2.7 to 3.7 m would reduce crash by 32%. Observations made in Denmark (1981) demonstrated that as the lane width increases, the relative crash frequency decreases: for road widths of fewer than 6 m, there was an increase in the risk of both injury crashes and severe injury crashes. This is supported by Serinivasan (1982) who reported that the crash rate of a 5 m road was about 1.7 time that of a 7.5 m road. A comprehensive Swedish study reported that, for roads with 90 km h-1 speed limits and similar alignments, increase in roadway width (carriageway plus shoulder) up to 13 m gives significant reductions in crash rates (Brude et al., 1980). However, a more recent Swedish work concluded that it was not possible to detect any statically significant differences in crash rates between wide and narrow roads (Bjorketun, 1982) of the three road-width classes used (8.5, 9 and 10 to 13 m), the 99 roads had a higher crash rate irrespective of the decade of construction.
Shoulder width: There have been a number of studies carried out on relationship between the shoulder width and crash rate. As noted by Hedman (1990), more recent studies show a decrease crashes with an increase in width from 0.0 to 2 m and little additional benefit is obtained above 2.5 m. However, Transportation Research Board (1978) concluded that, on multi-lane undivided and divided highways, shoulders that will not accommodate a parked vehicle off the travelled way increase the crash rate. As Transportation Research Board (1987) noted, the literature does not provide an entirely consistent model of the simultaneous effects of lane width and shoulder type on crashes. It also noted that crash rate decrease with increases in lane and shoulder width and that widening the lanes has a greater safety benefit than widening the shoulders.
Transition curves: Some studies have concluded that transition curves are dangerous because of the drivers’ underestimation of the severity of the horizontal curvature (Stewart and Cjfudworth, 1990; Simpson and Kerman, 1982). Stewart (1994) reports of a California Department of Transportation study involving a study of roads without transitions curves which showed that roads with transitions curves had, on average 73% more injury accidents (probability <1) than the others. Also the Department report Accidents on spiral Transition Curves in California warns against any use of these curves. However, it is understood that recent studies in Germany and the UK have concluded that the impact of transitions on safety is neural.
Gradients: Steep gradients are generally associated with higher crash rates. Hedman (1990) quoting Swedish’s research stated that grades of 2.5 and 4% increase crashes by 10 and 20%, respectively, compared with near-horizontal roads. Glennon et al. (1985) after examining the results of a number of studies in the United States concluded that grade sections have higher accident rates than level section; steep gradients have higher accident rates than mild gradients and down gradients have higher accident rates than up gradients. Department of Transportation (1981) included a graph related to the base accident rate to that on gradients which concurs with Glennon (1985) conclusions. Simpson and Kerman (1982) noted that the overall accident implications of steep gradients are not serving as it would appear first, since steep gradients have shorter length. Transportation Research Board (1978) concluded that the accident rate increases with gradients on curves.
Internal factors in horizontal alignment: Average crash rates are higher on horizontal curves than on tangent sections of rural 2-lane highways. Radius or degree of curvature consistently tops the list of geometry variables that most significantly affect operating speeds and crash experience on horizontal curves. Less consistent results concerning other geometry variables, including length of curve, defection angle, superelevation rate, presence of transition curves and the location of a curve relative to other horizontal curves, suggest that their effects may be statistically significant but lesser in magnitude.
Radius or degree of curvature: Many research efforts have identified radius or degree of curvature as a strong indicator of crash experience (Krammes et al., 1993; Glennon et al., 1985; Terhune and Parker, 1986). The mean radius and degree of curvature for each category were computed and regressed against the natural logarithm of mean accident rate within each category. The results support previous results that the sharpness of curve is significant. The high R results from the grouping of sites and therefore, does not reflect the variability among individual sites. Table 1 show the predication model developed from a Swedish study on road with 90 km h-1 speed limit (Brude et al., 1980).
Department of Transportation (1984) include graphs which compared crash rates for horizontal curvature to a base crash rate by means of a multiplier which agree closely with the Swedish values shown in Table 1. The difference between straight sections and bends becomes significant at a radius of about 1000 m. The UK data indicates continually increasing accident rate with reducing radius.
| Table 1: | Crash reduction factors for various increases in horizontal radii |
 | |
| *1 m = 3.28 ft. (Brude et al., 1980) | |
This increase in crash rate becomes particularly apparent at curve radii below 200 m. Simpson and Kerman (1982) noted that radius curves result in much shorter curve lengths and that the overall implications for accidents may be as it would appear.
It has been shown in past research that horizontal curves experience crash rates of up to 4 times the rates on tangent sections, all else being equal (FHWA, 2000; AASHTO, 1994; Zegeer and Deacon, 1987). Zegeer and Deacon (1987) identified the following components such as traffic, roadway and geometric features that influence safety at horizontal curve sections:
| • | Traffic volume on the curve and traffic mix (such as the percentage of trucks) |
| • | Curve features (such as degree of curve, curve length, superelevation, presence of transition curves) |
| • | Cross sectional curve element (such as lane-width, shoulder width, shoulder type, shoulder slope) |
| • | Curve section roadside hazard features (such as clear slope, rigidity and types of obstacles) |
| • | Stopping sight distance on curve (or at curve approach)z |
| • | Vertical alignment on horizontal curve |
| • | Distance to adjacent curves |
| • | Distance of curve to nearest intersection, driveway, etc. |
| • | Pavement friction |
| • | Presence and type of traffic control devices (signs and delineation) |
It has been shown by a number of researchers (Glennon et al., 1985; Zegeer et al., 1991; Glennon, 1987) that milder curves are associated with lower crash rates compared to sharper curves. For horizontal curves, casualty crashes seem to be more dominant than PDO (Property Damage Only) crashes. Those researchers also found that horizontal curves seem to have proportionately more head-on and opposite direction sideswipe crashes, fixed object, crashes, rollover crashes and night-time crashes compared to other sections. Glennon et al. (1985) and Fink and Krammes (1995) determined that the degree of curvature is the best predictor of crashes at curved sections. Geometric improvements used to improve safety at deficient horizontal curves include the following:
| • | Curve straightening |
| • | Roadway widening at curve sections |
| • | Superelevation improvements |
| • | Roadside improvements at curve sections |
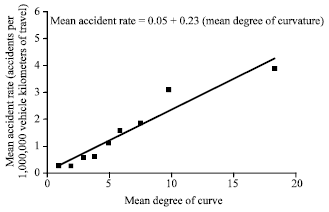 | |
| Fig. 1: | Relationship between mean crash rate and mean degree of curvature (Fink and Krammes, 1995) |
Also, traffic control devices are typically used to provide warning to curve approaches and to provide delineation of the pavement. These devices will be discussed in different section of this study. In their 1983 study, Glennon et al. (1985) developed a discriminate model to be utilized in identifying potentially hazardous horizontal curve sites on the basis of geometric, traffic and roadside features and conditions. The study found that hazardous roadside designs are the primary cause of crashes at horizontal curve sections. Fink and Krammes (1995) investigated the effect of degree of curvature, tangent length and sight distance on accident rates at horizontal curves and found that crash occurrence at curve sections was significantly influenced by the degree of curvature (Fig. 1). According to the researchers, variables not found statistically significant include preceding tangent length, sight distance, lane width, pavement width and condition.
Study by Zegeer et al. (1991) for the FHWA, the impacts of various geometric elements on crashes were investigated using a database comprising over 10,000 curve sections. The findings of this study were generally consistent with those of past studies as discussed earlier.
Superelevation: Horizontal alignment and superelevation of curves have an impact on the traffic safety performance of highway sections. Research that relates traffic safety to roadway horizontal alignment has consistently shown that traffic accidents increase with increasingly sharper curves. Sharp curves in segments that otherwise have good alignment, tend to surprise drivers and create even more hazardous situations. Consistency in design speeds along significant sections of highways has been advocated by some, as a means of controlling the incidence of surprise curves in other gentle alignments. However, design speeds for horizontal curves serve as functions of the maximum superelevation policies adopted by a design agency. Therefore, a single curve design may be regarded as having different design speeds by agencies that have different maximum superelevation policies (AASHTO, 2001).
The superelevation of horizontal curves is used as an input variable in the HSM methodology for rural two-lane highways. Superelevation is the pavement cross slope on the horizontal curve provided to counteract the tendency of vehicles to move toward the outside of the curve. As a measure of cross slope, superelevation is a ratio of two lengths and is therefore a dimensionless quantity, although many standard geometric design references assign it units of ft/ft. The HSM methodology considers the difference between the actual superelevation and the superelevation recommended by AASHTO policy. Superelevation affects safety in the HSM methodology only when this difference exceeds 0.01. Superelevation rates can be determined from existing data in computerized roadway inventory files, from as-built plans, or from field measurements Highway Safety Manual (HSM, 2008). This study summary of factors effective in crashes on the horizontal curves in two-line highways shows in the Table 2 classification.
Details of accident data: From data collected we selected seven main roads of two-lane highways in the province of Kohkilouyeh and Boerahmad in Iran. Crashes in the horizontal curves at 200 km in the regions Boerahmad (R4, 5, 6 and 7), Gachsaran, (R2) and Kohkilouyeh (R3), were investigated in terms of accident frequency in 2007. The findings of study about these roads can be generalized to Iran highways. The details of accident data have been collected and obtained from Traffic Safety Department of the traffic police, with information about each horizontal colligated curve. The collected data included number of accidents in the year 2007 and for each case, the cause of the accident, its time, weather, severity, types and location.
The present investigation incorporates examination of horizontal curve geometric design, design speed, singing, pavement, marking and road safety features. The selected horizontal curves include seven main roads of two-lane highways which were observed as explained below.
| Table 2: | Factors effective in crashes on the horizontal curves in two-line highways |
 | |
| Table 3: | Summary data main road- criteria for forming a horizontal curve in rural major arterial in K.B province of Iran |
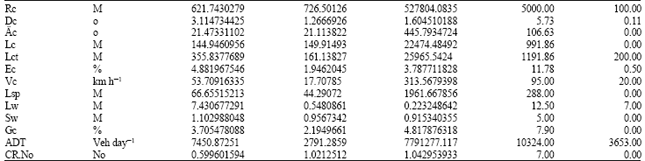 | |
The number of examined horizontal curves was 502 and each variable, included Radius curve (Rc), Degree curve (Dc), Delta curve (Δc), Length curve (Lct), lane width 3.5 meter per lane, left and right clearance width 1.0 and 0.0 m regularity, signing (include advance, warning, directional that are provided), road marking provided with deficiency, road condition as pavement surface in good condition with main road lighting and observations such as high travelling speed of vehicles over curves more than 50 km h-1, Table 3 shown summary Data Main Road-Criteria for Forming a Horizontal Curve in Rural Major Arterial in K.B province of Iran.
R Software: The required data about 2 line rural (highways) will be collected from Iranian Highway Police headquarter. Then data will be entered into R software statistical package version 3.0 (USA) for descriptive statistical analysis.
RESULTS AND DISCUSSION
The relationship between horizontal curve factors and crash rates is quite complex and not fully understood. Relatively little information is available on the relationships between many geometric elements and crash rates, although it has been clearly shown that very restrictive geometric elements such as very short sight distances or sharp horizontal curve in considerably contribute to higher crash rates and that certain combination of element cause an unusually severe crash problems.
| Table 4: | Statistical summary of poison regression model |
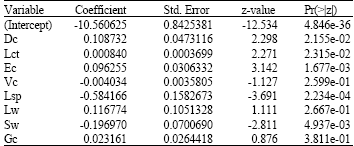 | |
No. of observations in the fit: 502; Degrees of Freedom for the fit: 9; Residual Deg. of Freedom: 493; Cycle:2; Global Deviance: 1012.997; AIC: 1030.997; SBC: 1068.964; GAMLSS-RS iteration 1: Global Deviance = 1012.997; GAMLSS-RS iteration 2: Global Deviance = 1012.997 | |
However, it appears that significant reductions in the values of some of the elements specified in geometric design standards do not result in large increases in crash rates. There is broad agreement on the general relationship between geometric design elements and crash rates. Consequently, for the purposes of evaluating safety impacts of lower physical design standards or for comparing the safety of alternative road alignments, the available information should provide a reasonable indication of the likely differences in expected crashes.
Table 4 shows the results from Poisson regression model that an increase in independed variables of degree curve, length total curve, superelevation curve and offset variable ADT for horizontal curves leads to an increase in the number of crashes and an increase in independed variables length spiral curve, shoulder width ends up with a decrease in the number of crashes.
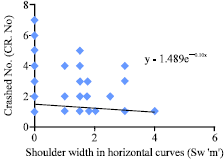 | |
| Fig. 2: | Relationship Sw and CR.No |
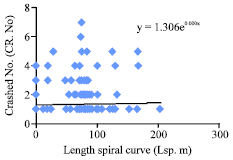 | |
| Fig. 3: | Relationship Lsp and CR.No |
According to the parameter estimates obtained in this model, the model form can be written as in Eq. 2:
| (2) |
| CR.No | = | No. of horizontal curve-related crashes, |
| ADT | = | average daily traffic (veh day-1) |
| Dc | = | Degree horizontal curve (°) |
| Ec | = | Superelevation horizontal curve (%) |
| Lsp | = | Length spiral curve (m) |
| Sw | = | Shoulder Width (m) and |
| Lct | = | Total length segment of horizontal curve (m), equal (two times length spiral plus length horizontal curve) |
The coefficients of full model show that shoulder width has negative sign, signifying that for a unit increasing in total shoulder width (Fig. 2) and a unit increasing in length spiral curve the car-related crashes decrease (Fig. 3) constantly by considering the effect of other variables. Also with increasing in the degree curve, average daily traffic and superelevation, crashes rate increases (Fig. 4, 5). The other variables which include grade curve, line width and limited speed in horizontal curve are over than Pr (>|z|, z=.05).
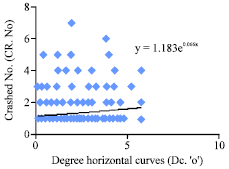 | |
| Fig. 4: | Relationships Dc and CR.No |
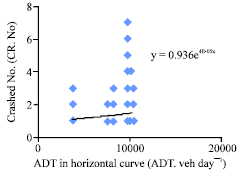 | |
| Fig. 5: | Relationship ADT and CR.No |
That rejects in model. Relationships between number of crashes and Horizontal Curve Elements are shown in the Fig. 2-5.
The result in this study shown with decrease in horizontal curve degree, causes horizontal curve radius increase. And increase in horizontal curve radius generally yields sufficiently among crash reduction. That concurs with founds of (Krammes et al., 1993; Glennon et al., 1985; Terhune and Parker, 1986; Brude et al., 1980; Fink and Krammes, 1995; Labi and CATS, 2006).
Result this study shown with in this model, gradient and speed, indicator variable which indicates the horizontal curves is found to be insignificant and is not included in the model. Whereas, Vavilikolanu (2008) showed that by flattening steep curves, the safety on vertical curves may increase. And also showed an increase in truck-related crashes as posted speed limits increase. This may be explained as the speed of the vehicle increases, sight distance increases and the vehicle travels further in the direction.
There have been a number of studies carried out on relationship between the shoulder width and crash rate that concurs with found this study that increases shoulder width in two line highway crashes rate decreases include Transportation Research Board (1978, 1987) and Hedman (1990).
There are many influential factors in traffic crashes which are taken as the criteria for highway safety. Road horizontal curve elements are among these effective factors. As the relationships between highway safety and road horizontal curve elements are considered some relationships can be seen intuitively at first approach. However, the important point is to determine the level of these relationships quantitatively. Although, the relationships show the same tendency, their level vary according to each country`s characteristic conditions.
It was realized in this study that elements related to horizontal curve geometry are more effective on road safety than elements with road geometry. The other influential elements include-according to their importance-cross section elements, vertical geometry, roadside features, traffic volume and sight distances. The study of the relationship between geometric design and safety yielded the following conclusions:
The most important independent effective variables in the horizontal curves crashes based on the analysis of the collected data are: degree of the horizontal curve (Dc), total length segment of horizontal curve (Lct), superelevation of the horizontal curve (Ec), length of the spiral curve (Lsp), shoulder width (Sw) and offset variable average daily traffic (ADT).
Horizontal curves are more dangerous when combined with gradients and surfaces with low coefficients of friction. Horizontal curves have higher crash rates than straight sections of similar length and traffic composition; this difference becomes apparent at radii less than 1000 m. The increase in crash rates becomes particularly significant at radii below 200 m. Small radius curves result in much shorter curve lengths and overall implications for crashes may not be as severe as would first appear.
Recommend continued use of 4, 6, 8, 10 and 12% maximum rates. Promote design consistency with an area of similar climate and character. Develop the minimum radii with normal crown for each of the five maximum superelevation rates. Only presents the minimum radius for a 1.5% normal crown and a maximum superelevation rate of 10%.
There is only a minor decrease in the speed adopted by drivers approaching curves of radii which are significantly less than the minimum radii specified for the design speed. However, curve radii below 200 m have been found to limit the mean speed to 90 km h-1.
Crashes increase with gradient and down-gradients have considerably higher crash rates than up-gradients. However, the overall crash implications a steep gradients may not be severe since steeper gradients are shorter. The geometry of horizontal curves is not known to have a significant effect on crash severity.
There appears to be little erosion of safety resulting from the use of sight distances below the minimum values specified in geometric design standards, although there is a significant increase in the crash rate for sight distance below 100 m.
As the lane width increases above minimum, the crash rate decreases. However, the marginal rate diminishes with increased lane width. On multi-lane highways, the more lanes that are provided in travelled way, the lower the crash rate.
Shoulders wider than 2.5 m give little additional safety benefit. As the median shoulder width increases, crashes increase. The present of a median has the effect of reducing specific type of crashes, such as head-on collisions. Medians, particularly with barriers, reduce severity of crashes.
Most of the than recent studies emphasize on the impact of transition curves in the horizontal curves crashes and recommend use of transition curves with length curve than 75.0 m.
Methods for improving the safety of horizontal curves include: (1) reconstructing the curve to make it less sharp, (2) widening lanes and shoulders on curves, (3) adding spiral transitions to curves, (4) increasing the amount of superelevation (up to allowable maximums of 0.80 and1.0 in urban and rural areas, respectively), (5) increasing the clear roadside recovery distance by relocating utility poles and trees, (6) improving vertical and horizontal alignment by avoiding sharp left hand curves and sharp downgrades, (7) assuring adequate pavement surface drainage on long radius curves and location where cross drainage is longer than on lane in width and (8) providing increased surface skid resistance on downgrade curve sites.
ACKNOWLEDGMENTS
We would like to thank the Sustainable Urban Transport Research Centre/Department of Civil and Structural Engineering, Faculty of Engineering and Built Environment, Universiti Kebangsaan Malaysia, 43600 UKM, for supporting the related studies. Special thanks to Iran Transportation and Terminals Organization (ITTO) for their earnest cooperation and also to the Kohgilouyeh and Boyerahmad State Police which provided us with their annual reports which formed our crash database. I also would like to express my gratitude to all corporations and agencies who contributed to this study with their maps, studies and highway and transportation consultations, especially those Iranian corporations such as ETHAD RAH, BRAYAND, PARSELO, IRAN ASTON and other companies which cooperate with Road and Transport Department of Kohgilouyeh and Boyerahmad province.
REFERENCES
- Simpson, D. and J.A. Kerman, 1982. The research and development background to highway link design. Traffic Eng. Control, 23: 414-421.
Direct Link - Stewart, D. and C.J. Cjfudworth, 1990. A remedy for accidents at bends. Traffic Eng. Control, 31: 88-93.
Direct Link - Transportation Research Board, 1978. Cost and safety effectiveness of highway design elements. TRB, pp: 237.
Direct Link - Yager, M. and R. Van Aerde, 1983. Geometric and environment effects on speed of 2-lane highways. Trans. Res. Part A: General, 17A: 315-325.
CrossRef









umar junaidu ningi Reply
looking for sample of journal on redesign horizantal alignment using mx-road application
umar junaidu ningi Reply
the abstract where perfect in the curve where suitable for all design horizontal and vertical alignment of the road design.