Research Article
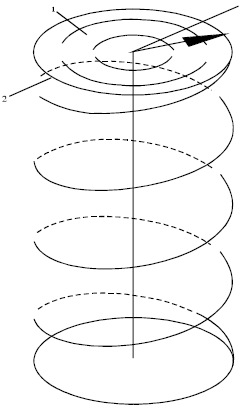
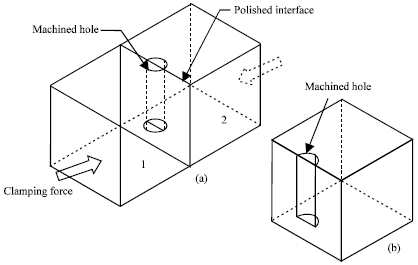
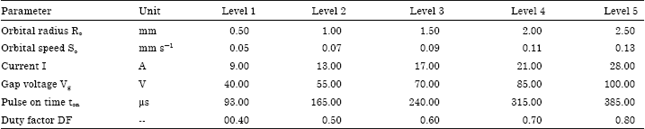
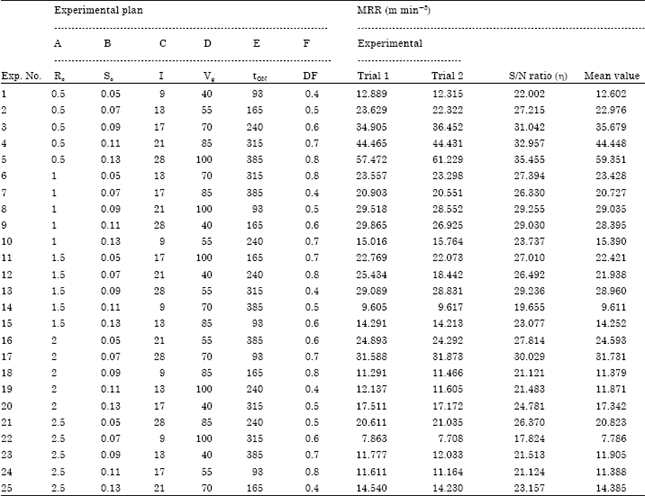
Modeling and Analysis of Material Removal Rate During Electro Discharge Machining of Inconel 718 under Orbital Tool Movement
Department of Mechanical Engg., S.V. National Institute of Technology, Surat, India
Keyur P. Desai
Department of Mechanical Engg., S.V. National Institute of Technology, Surat, India
Harit K. Raval
Department of Mechanical Engg., S.V. National Institute of Technology, Surat, India