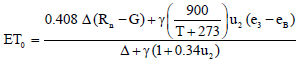Research Article
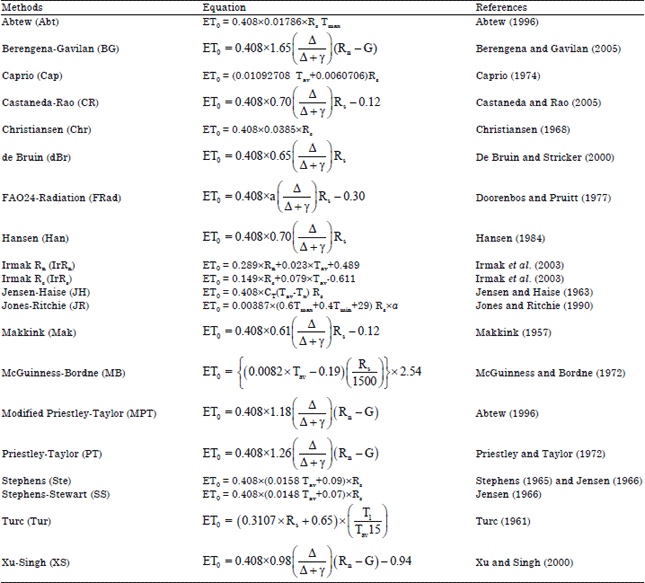
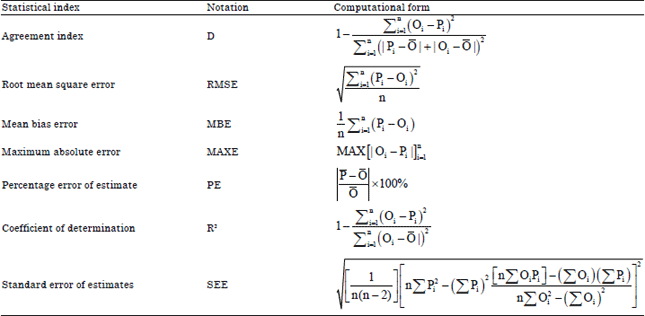
Comparative Performance of Reference Evapotranspiration Equations at Sub-Humid Tarai Region of Uttarakhand, India
Department of Irrigation and Drainage Engineering, College of Technology, Govind Ballabh Pant University of Agriculture and Technology, Pantnagar, Uttarakhand, 263145, India