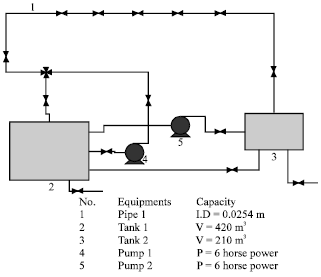
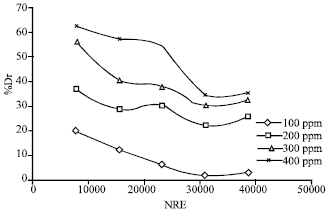
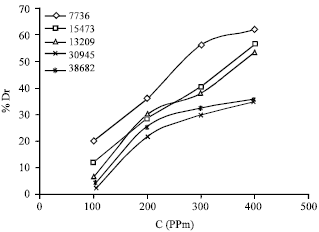
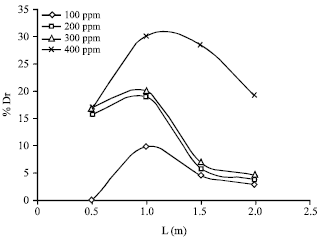
Research Article
Drag Reduction Characteristics Using Aloe Vera Natural Mucilage: An Experimental Study
Faculty of Chemical and Natural Resources Engineering, University Malaysia Pahang, Gambang, 26300, Kuantan, Pahang, Malaysia
Kumaran Letchmanan
Faculty of Chemical and Natural Resources Engineering, University Malaysia Pahang, Gambang, 26300, Kuantan, Pahang, Malaysia
Rosli Mohd. Yunus
Faculty of Chemical and Natural Resources Engineering, University Malaysia Pahang, Gambang, 26300, Kuantan, Pahang, Malaysia