Research Article
Pathogens as Biological Tools in Host Competition
Department of Ecology and Evolution, 321 Steinhaus Hall, University of California, Irvine, CA 92697, USA
Most living organisms are susceptible to pathogens. Many infections have a detrimental effect and reduce the fitness of the host (Neuhaus, 2003). To counter pathogens, organisms have evolved defense strategies and immune systems (Muller et al., 1999; Kimbrell and Beutler, 2001; Rolf f and Siva-Jothy, 2003). If an infection is associated with a host, improved immunity and lower pathogen prevalence will result in an increased host fitness, subject to tradeofs (Brown, 2003; Sandland and Minchella, 2003). Thus, we see a coevolutionary race between pathogen infectivity and host resistance (Frank, 1991; Anderson, 1995; van Baalen, 1998; Boots and Bowers, 1999; Frank, 2000). This argument, however, considers a single host species in isolation. In the natural world, different host species interact with each other in a number of ways. In this context, the concept of apparent competition becomes important (Holt, 1977). That is, competition between two host species can be mediated through a shared parasite, even if the hosts do not interact directly. If the infection is less costly to one host species relative to another, this can result in “competitive exclusion”. The species which survives is the one which can support higher pathogen loads in the population. Apparent competition has been studied theoretically (Holt, 1977; Greenman and Hudson, 1999, 2000), experimentally (Hassell and Bonsall, 1997) and in the context of field studies (Hudson and Greenman, 1998; Tompkins et al., 2000; Tompkins et al., 2001). The aim of this study was to discuss this research in a different light. That is, from the viewpoint of the immune system and its evolution. This study a brief overview and discuss how the recovery rate from infection (and thus immunity) research could determine the fitness of hosts in the context of apparent competition. Then review recent research which has explored this topic with a mathematical model. Finally, discussed specific field studies in the light of this work.
Apparent Competition and Recovery from Infection
Theoretical work on apparent competition suggested that it could be an important factor responsible for structuring ecological assemblages (Holt, 1977). This has been confirmed by experiment using a laboratory insect system (Hassell and Bonsall, 1997). Two host species shared a common parasite but were not allowed to interact directly. While both single host-parasitoid interactions were stable in separation, one of the species was excluded when the parasitoid was shared. Several examples of species exclusion in the wild have been attributed to apparent competition, where a common parasite infects two host species and causes different degrees of pathogenicity (Hudson and Greenman, 1998; Tompkins et al., 2000, 2001; Tompkins et al., 2002). The host species in which the parasite is less pathogenic could exclude the host in which the parasite is more pathogenic. Specific examples will be discussed below. The empirical research was coupled with further theoretical explorations. Mathematical models were constructed which included both direct and apparent competition (Greenman and Hudson, 1999, 2000). Moreover, parameter estimations (Tompkins et al., 2000) supported the hypothesis that shared parasites can be responsible for determining patterns of species decline and exclusion observed in the wild.
This study has obvious implications for the role of disease recovery for inter-specificc competition and thus for the evolution of immune efficacy.
Does it pay to maintain suboptimal immune responses such that a parasite can be used as a tool to fight a competitor? To examine this question, we have to consider an extended model for apparent competition. So far, most of the literature has considered a single parasite which is shared among hosts. To study the role of recovery from infection in more detail, it makes sense to study a system where two host species share two parasites. Each host species has a native pathogen to which it is adapted. The native pathogen can, however, also infect the other host species (cross-species infection) in which it can have increased virulence. This scenario has been captured recently in a mathematical model (Wodarz and Sasaki, 2004) which we review in the following sections. We will discuss a basic ecological model, include space, and examine evolutionary processes.
In this context, it is important to point out that we consider two levels of competition. First, we examine competition between host species (inter-specificc competition).For the evolutionary treatment, we also consider intra-specific competition: That is, a host species consists of different variants which different in their recovery rate from infection. Because the variants of a given species share habitat and food, they compete against each other.
Basic Inter-specific Competition Dynamics
We start by considering a simple model which captures the competition between two host species and the effect of cross-species infection. Both host species carry a native pathogen. Upon exposure, the native pathogen of one host species can, however, also infect the other host species. Thus, four populations are considered, i.e., host species 1 and its native pathogen and host species 2 and its native pathogen. The model is explained schematically in Fig. 1 and the mathematical approach is explained in the Appendix. The effect of cross-species infection is treated as follows. Pathogen 1 can infect host species 2 and pathogen 2 can infect host 1. It is further assumed that this cross-species infection results in more virulence than infection with the native pathogen (because there is lack of adaptation). In fact, we assume an extreme scenario for simplicity: Cross-species infection kills the competitor and the competitor cannot pass on the pathogen. In model terms, cross-species infection kills the host population with a defined rate. The assumption that cross-species infection results in higher degrees of virulence is supported by several field studies which will be discussed in detail below (Tompkins et al., 2000, 2001, 2002). Patterns of virulence in the context of multihost pathogens can, however, vary (Woolhouse et al., 2001). In cases where our assumption does not hold, our analysis does not apply.
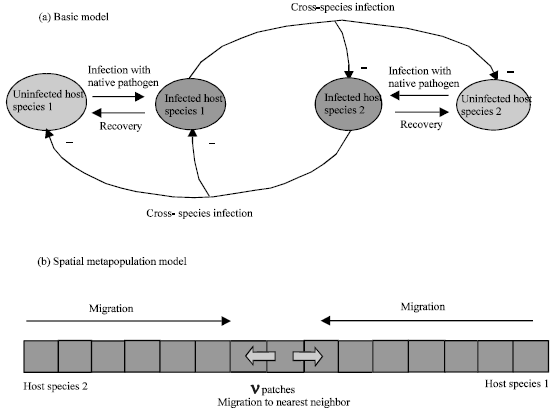 | |
| Fig. 1: | Schematic representation of the mathematical models. (i) The basic model. It is described in the text and in Box 1. (ii) The model is extended as a metapopulation model to include space and migration |
In the following we point out the biologically interesting insights gained from this model. We are interested in how the rate of recovery from infection influences the competitive ability of the hosts. Hence, we assume that the two host species vary in this parameter. For simplicity, we assume that all other parameters are identical for the two host species and that they are competitively neutral in the absence of pathogens. This simplification is valid because it concentrate on the effect of apparent competition. In the presence of the pathogens, the following two outcomes were observed.
| • | Single species endemic equilibrium: One species survives and out competes the other. The native pathogen is maintained in the population. Thus, there are two such equilibria, depending on which species wins. |
| • | Coexistence equilibrium: Both species coexist and the respective pathogens are maintained. |
The recovery rate from infection for the two host species influences the outcome of the dynamics (Fig. 2). Coexistence of the two host species is only possible in a limited parameter region when the recovery rates of both species are low. Otherwise, we observe the following pattern. If the recovery rate of species 1 lies below a threshold relative to that of species 2, species 2 wins. The cost of carrying the pathogen in the population outweighs the benefit. If the recovery rate of species 1 is high and lies above a threshold relative to species 2, species 2 wins again.
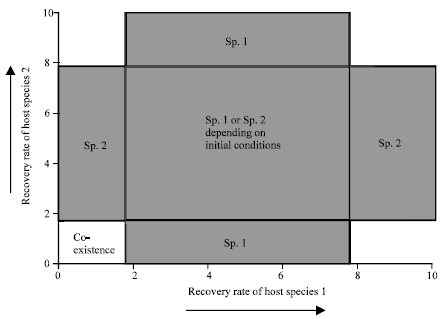 | |
| Fig. 2: | The effect of the recovery rate from infection on the competition dynamics of two host species (Sp. 1 and Sp. 2)which share two species of parasites. The thresholds separating the different parameter regions have been calculated from expressions derived from the mathematical model. They depend on parameters which determine the basic reproductive ratio of the pathogens and on the degree of host mortality upon cross-species infection. The parameter regions which are left blank represent outcomes which are not relevant for the present context and which are not discussed in this paper. Parameters were chosen as follows. βi = 1; ρi = 0.1; qi = 0.1; ri = 0.5; di = 0.1; αi = 0.1; bi = 0.1 |
This is because a high recovery rate keeps the pathogen at low levels, allowing for only little transmission to the competitor (species 2). On the other hand, pathogen transmission is more efficient from species 2 to species 1 and this asymmetry explains that species 2 wins. If the recovery rate of species 1 is intermediate (between the thresholds cited above), it can win and outcompete species 2. The pathogen is prevalent enough to be used as a biological tool, while it is not too costly for the native host. If the recovery rates of both species are intermediate (parameter space including the orange to blue gradient in Fig. 2), then two outcomes are stable (bistability): either species 1 wins, or species 2 wins, depending on the initial conditions. The higher the initial abundance of one species relative to the other, the more likely this species is to win and persist. In this region of bistability, the exact initial abundance of the host species required to win the competition depends on the recovery rate from infection. The higher the recovery rate, the higher the initial abundance required to outcompete the other host species. This is because a higher recovery rate compromises the use of the parasite as a tool in host competition. Mathematical conditions which defined thresholds and parameter regions are defined in Wodarz and Sasaki (2004).
The Effect of Space
Here we concentrate further on the parameter region where the outcome depends on initial conditions. Including space and migration into the model gives rise to further interesting results. Space can be formulated as a metapopulation model (Fig. 1b)(Wodarz and Sasaki , 2004).We consider a one-dimensional space which comprises v patches along which uninfected and infected hosts can migrate. In each patch, local dynamics occur as described in the basic competition model explained in the last section.
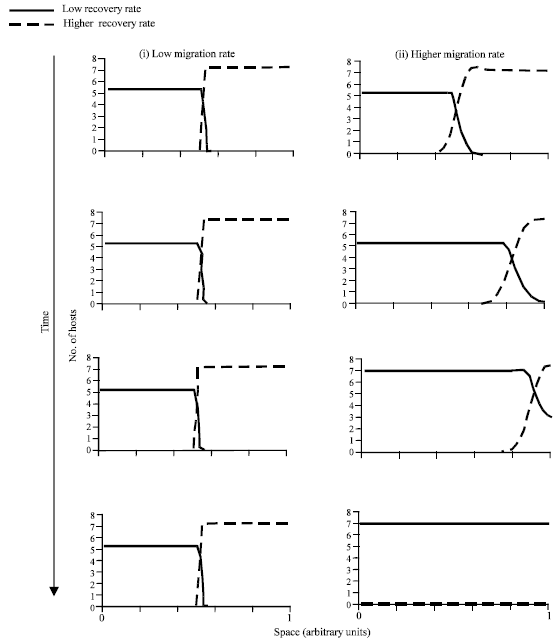 | |
| Fig. 3: | Outcome of the competition dynamics in a spatial setting. The sequential graphs depict how the spatial distribution of the two host species develops over time. (I) Dynamics assuming a low migration rate. The graphs show an outcome in which the two host species coexist across space. That is, they cannot invade each others’ territories. (ii) Higher migration rate. The species with the lower recovery rate invades the territory of its competitor and excludes it. Parameters were chosen as follows. βi = 1; ρi = 0.1; qi = 0.1; ri = 0.5; di = 0.1; αi = 0.1; bi = 0.1; γ1 = 7; γ2 = 5; m = 0.000001 for lower migration rate; m = 0 .0001 for higher migration rate |
In addition, migration of hosts occurs to the nearest neighboring patches at a rate (m). As starting conditions we assume that species 1 is at equilibrium at one boundary, while species 2 is at equilibrium at the other boundary. We study the dynamics of interspecifc competition in dependence of the migration rate (m) (Fig. 3). In the present context, we distinguish between two parameter regions, depending on the value of m. (i) If the migration rate (m), is low and lies below a threshold, the two species meet in the middle of the one-dimensional space, but cannot invade each others territory. In other words, they coexist across space. The reason is as follows. A low migration rate results in a low initial abundance of the invading species relative to that of the resident species in the patch occupied by the resident species. Under such initial conditions, invasion cannot occur. (ii) If the migration rate (m), crosses the threshold, we find that the host species with the lower recovery rate invades the territory of its competitor and excludes it. This is because a higher migration rate ensures that the initial population size of the invading species is now higher relative to that of the resident species in the patch of the resident species. This larger invasion force tips the balance in favor of the invading species. As evident from this discussion, the migration rate determines the initial abundance of an invader relative to the resident. The migration rate threshold therefore has the same properties as the initial condition threshold discussed above. Thus, the higher the recovery rate of the superior competitor, the lower its competitive advantage and the higher the migration rate threshold required for invasion.
Evolutionary Dynamics
As discussed above, a species with a lower recovery rate can out compete a species with a higher recovery rate because the relative fitness of the competitor is reduced by cross-species infection. Here we ask in which direction the host recovery rate is expected to evolve. We start with the spatial model described above. In addition, we assume that in each species, mutation can give rise to new variants that differ in their recovery rate. The different mutants of a given species are assumed to be in competition with each other. Thus, there is both intra-and inter-specific competition. Intra-specific competition occurs between variants of a single species, while inter-specific competition describes the interactions between the two host species. Within a given species, it was found that a mutant with an increased recovery rate can always invade and outcompete a variant with a lower recovery rate. Thus, evolution will take a species towards stronger immunity until the recovery rate crosses a threshold and the pathogen cannot be maintained in the population anymore (the basic reproductive ratio of the pathogen is less than one). Therefore, evolution leads to a loss of parasites. This can have the following consequences. If evolution increases the recovery rate of a given species, it can decrease its fitness relative to that of a competitor. Assume that evolution increases the recovery rate of species 1 beyond a threshold relative to species 2. Now, species 2 can invade the territory of species 1 and drive it extinct (Fig. 4). Thus, evolution can take the species into a parameter region in which it is prone to competitive exclusion. While a lower recovery rate gives a species an advantage over a competitor, a higher recovery rate is selected for within a species. In other words, intraspecific competition dominates over inter-specific competition. The exact recovery rate threshold beyond which invasion by the competitor is observed is hard to defined (Wodarz and Sasaki, 2004). If the recovery rate is in the parameter region of bistability (Fig. 2), then the outcome will depend on the migration rate of the hosts as explained above. If the recovery rate is higher and in the parameter region where the only outcome is exclusion, then invasion will always happen.
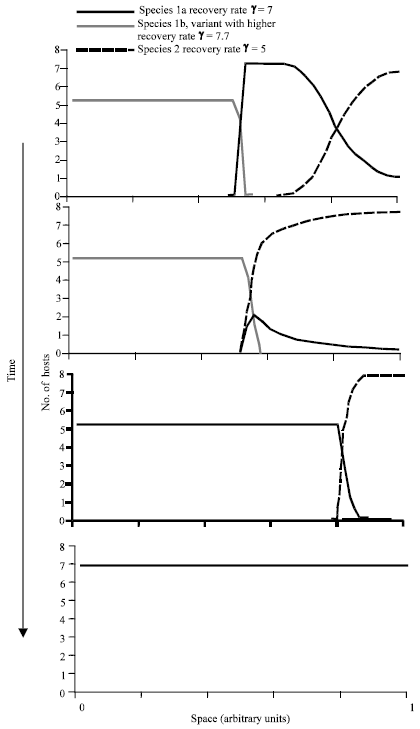 | |
| Fig. 4: | Evolution of the recovery rate from infection. We assume that there are two variants of species 1 which differ in the recovery rate. Species 1a has a lower recovery rate than species 1b. Both variants of species 1, however, have a higher recovery rate that species 2. Species 1a can coexist with species 2 across space. However, species 1b outcompetes species 1a. Because species 1b has an elevated recovery rate from infection, it can then be excluded by species 2. Parameters were chosen as follows. βi = 1; ρi = 0 .1; qi = 0 .1; ri = 0 .5; di = 0 .1; αi =0 .1; bi = 0 .1; m = 0 .0001 |
Theory and Field Studies
In this section, we recapitulate the main insights gained from the above discussion and then discuss implications for specific field studies in detail. The main points regarding the relationship between apparent competition and the role of immunity are as follows.
| • | The basic ecological dynamics suggest that a relatively low rate of recovery from infection can give a host species a competitive advantage over a species with a higher recovery rate. While the parasite burden is costly for the host, the ability to transmit the parasite to the competing species and hence to damage the competitor, can outweigh this cost. Therefore, a higher parasite burden allows the pathogen to be used as a biological tool in host competition. |
| • | The spatial model introduces new aspects to these dynamics. For low migration rates, it allows for coexistence of the two host species across space. The pathogen serves a defensive role and prevents invasion by the competitor. For higher migration rates, the host with the lower recovery rate drives its competitor extinct. The pathogen serves a defensive role and enables the host to invade new space. |
| • | The evolutionary model suggest that evolution maximizes the recovery rate from infection and minimizes parasite burden. This is because intra-specific competition is more important than inter-specific competition. Hence, evolution takes the species into a parameter region in which it is prone to be excluded by a competitor. |
This theoretical discussion has important implications for understanding observed patterns of species exclusion or coexistence in the wild. The most basic message is that the transmission of parasites across species may have to be taken into considerations in order to explain the outcome of competition dynamics. This conclusion has also been reached in a number of previous studies (Hudson and Greenman, 1998; Greenman and Hudson, 1999, 2000).
Perhaps the best studied example is the competition between red squirrels (Sciurus vulgaris )and grey squirrels (Sciurus carolinensis) in the UK (Tompkins et al., 2002). When grey squirrels were introduced to the UK they invaded the territory of the resident red squirrels which consequently declined. Experimental data suggest that the replacement of red squirrels by grey squirrels could be caused by a parapoxvirus which was introduced to the UK together with the grey squirrel. Experiments have shown that parapoxvirus infection is highly pathogenic in red squirrels, whereas it does not have any detectable effect on the health of grey squirrels. In support of this hypothesis, grey squirrel seroprevalence to parapoxvirus correlates with the degree of decline of the red squirrel populations. The decline of red squirrels is most marked in English and Welsh populations, where grey squirrel seroprevalence to parapoxvirus is high. On the other hand, the negative impact of red squirrels is least apparent in Scottish and Irish populations where seroprevalence is zero.
Another intriguing example is the interaction between the ring-necked pheasant Phasianus colchicus and the grey partridge Perdix perdix (Tompkins et al., 2000, 2001).The pheasant population maintains an infection with the caecal nematode Heterakis gallinarum. While this parasite cannot be maintained in the partridge population because of low reproductive potential in this species (Tompkins et al., 2000), the pheasants can infect the partridges in which the infection is significantly more virulent. Empirical and theoretical studies suggest that this parasitic infection could be responsible for the decline of wild grey partridge populations in the UK which has been observed over the past 50 years (Tompkins et al., 2000).
These examples however, represent situations in which a single parasite is shared between two species of hosts. One of the species carries the pathogen. It serves to fight the second species which, however, cannot retaliate in the same way. Therefore, these dynamics are asymmetric and are expected to result in the exclusion of one species. In the wild, however, exclusion of species is a relatively rare event. If both host species carry pathogens which can affect the balance in competition, the mathematical models suggest that both exclusion and coexistence can be possible, depending on the spatial setting and the migration rates of the species. If the migration rate is low, we can expect to observe that two species coexist and remain confined to a fraction of a given habitat, although they could in principle colonize the whole area. The fact that space can promote coexistence is a well known ecological result (Bonsall and Hassell, 2000). The reason for this behavior in our model is that mutual cross-species infection prevents host species from invading their respective territories. So far, detailed field or experimental studies addressing such dynamics have not been performed.
A more complex example which comes closer to the two-hosts two-parasite situation is the transmission of blood parasites between resident and migratory song birds. Waldenstrom et al. (2002) studied the phylogeny of avian haemosporidian parasites, Haemoproteus and Plasmodium, in a number of African resident and European migratory song bird species. It was shown that host sharing and cross-infection was common both in Haemoproteus and in Plasmodium. The phylogenetic analysis indicated that transmission occurred both in Africa and Europe. Infection with these parasites can result in significant fitness costs, especially when hosts are exposed to lineages for the first time and are not adapted to them. Therefore, resident birds in Africa may act as reservoirs for tropical avian blood parasites. These parasites can infect foreign migrants when reaching their African winter quarters and reduce their fitness. Thus, resident species of songbirds benefit from carrying the parasites because invasion of their habitat is costly for the migratory bird species. This could put pressure on the migrating species to select wintering habitats which do not significantly overlap with the territories of related resident bird species. Similarly, the migratory birds could carry parasites which can be transmitted to the resident species and help in the colonization of the wintering habitats.
This study has explored the relationship between the recovery rate from infection and the competitive ability of hosts. In the context of inter-specific competition we found that an intermediate or relatively low recovery rate from infection can give a host species an advantage over its competitor. In a spatial setting, the dynamics can result in a variety of outcomes. These include failure of invasion and thus coexistence of different host species in separate territories; or successful invasion and exclusion by a competitor characterized by less efficient immunity. We have discussed field data which show how cross-species infection can modulate host competition in relatively simple settings. In the light of theory, it would be interesting to perform more detailed ecological and epidemiological studies to examine the effect of cross-species infection on parasite-host dynamics. The role of parasites as biological tools should not only be considered to explain species exclusion, but also to explain patterns of species coexistence and separation of habitats.
A particular application of these studies in the context of human health are zoonotic infections. Over 60 percent of pathogenic human infections have been found to be zoonotic (Taylor et al., 2001) and zoonotic infections are thought to be associated with emerging diseases (Daszak et al., 2000; McCarthy and Moore, 2000). Zoonotic infections include many different types of parasites, such as viruses, bacteria, protozoa and helminths. Among viruses, prominent examples are ebola, influenza and human immunodefficiency virus. In general, zoonotic infections emerge in humans following growth of the human population, mobility and migration of humans and increased intrusion into the habitats of animals species (Mahy and Brown, 2000). In the context of humans it is unclear whether infections have ever prevented expansion and invasion of new territories. Among animal populations, ebola virus poses a threat to the survival of great apes (Vogel, 2003; Walsh et al., 2003). The reservoir and epidemiology of ebola virus is not clear, but rodent or other reservoirs have been postulated. More practical examples involve livestock. Infections with wild-life reservoirs (e.g., rinderpest or trypanosomes) have had a substantial impact on land use in sub-Saharan Africa (Barrett and Rossiter, 1999; Rweyemamu et al., 2000; Aksoy et al., 2003). These examples underscore the notion that wildlife parasites which can be transmitted to human and animal populations can render habitat invasion more difficult because increased virulence upon cross-species infection leads to a significant reduction in host fitness.
Appendix: The Basic Mathematical Model
We consider two host species which utilize the same resource and two pathogens specialized to each host species. We assume that when cross-species infection occurs, the pathogen kills the foreign host, but cannot become infectious in it. Let xi and yi be the susceptible and infected (and infectious) host density of species i (i =1 , 2), which change with time as
| (1) |
| (2) |
| (3) |
| (4) |
where bi, di and hi are the birth rate, the natural mortality and the coefficient of density dependent mortality of host species i ; βi , γi and αi are the transmission rate, the recovery rate and the virulence (additional mortality) of pathogen species i in its native host. The host becomes susceptible when it is recovered from the infection (SIS model) and the infected host cannot reproduce. pi and qi are the rates at which susceptible and native-pathogen-infected hosts are cultivated by the cross-species infection. For mathematical details (Wodarz and Sasaki, 2004).
In order to concentrate on the effect of apparent competition, we consider a simplified scenario where the host species only differ in the recovery rate from infection. The host species are therefore competitively neutral in the absence of pathogens. This is very unlikely to be true in nature. The simplification, however, allows us to discuss results in relatively easy terms. At the same time, relaxation of this simplification does not change the findings about the role of parasites in host competition which are reviewed here. This model forms the basis for the discussion in this review. For further details and extensions, including space and mutations (Wodarz and Sasaki, 2004).