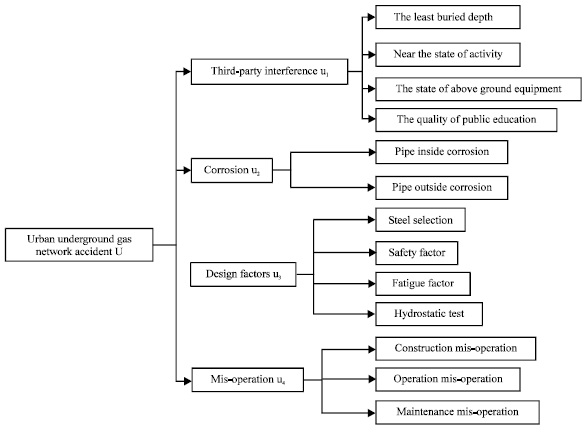
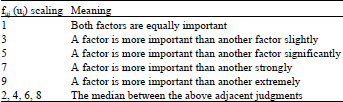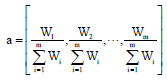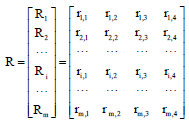Research Article
Risk Assessment of Urban Gas Pipeline Network Based on Fuzzy Multi-attribute Method
China University of Petroleum, Beijing, 102249, Beijing, People`s Republic of China
Jianchun Fan
China University of Petroleum, Beijing, 102249, Beijing, People`s Republic of China
Wei Zhu
Beijing Key Laboratory of Operation Safety of Gas, Heating and Underground Pipelines, Beijing Research Center of Urban Systems Engineering, 100089, Beijing, People`s Republic of China
Yongqiang Bai
Beijing Municipal Institute of Lahour Protection, 100054, Beijing, People`s Republic of China