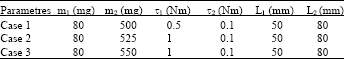Research Article
Dynamic Modeling and Simulation of Ionic Polymer Metal Composites (IPMC) Actuated Manipulator
College of Mechanical Engineering, Harbin Engineering University, 145-1 Nantong Street, Nangang, Harbin, 150001, Heilongjiang, People`s Republic of China
Zhao Gang
College of Mechanical Engineering, Harbin Engineering University, 145-1 Nantong Street, Nangang, Harbin, 150001, Heilongjiang, People`s Republic of China
Tran Linh Khuong
College of Mechanical Engineering, Harbin Engineering University, 145-1 Nantong Street, Nangang, Harbin, 150001, Heilongjiang, People`s Republic of China
Zhuang-Zhi Sun
College of Mechanical Engineering, Harbin Engineering University, 145-1 Nantong Street, Nangang, Harbin, 150001, Heilongjiang, People`s Republic of China