Research Article
Simulation of Phenol Adsorption in a Packed Bed Column
Chemical Engineering Programme, Faculty of Engineering, Universiti Malaysia Sabah, 88400 Kota Kinabalu, Sabah, Malaysia
S.M. Anisuzzaman
Chemical Engineering Programme, Faculty of Engineering, Universiti Malaysia Sabah, 88400 Kota Kinabalu, Sabah, Malaysia
C.G. Joseph
Water Research Unit, Faculty of Science and Natural Resources, Universiti Malaysia Sabah, 88400 Kota Kinabalu, Sabah, Malaysia
S. Abang
Chemical Engineering Programme, Faculty of Engineering, Universiti Malaysia Sabah, 88400 Kota Kinabalu, Sabah, Malaysia
L.C. Siang
Chemical Engineering Programme, Faculty of Engineering, Universiti Malaysia Sabah, 88400 Kota Kinabalu, Sabah, Malaysia











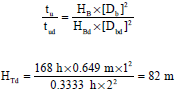
Shoaib Ahmed Reply
Hi,
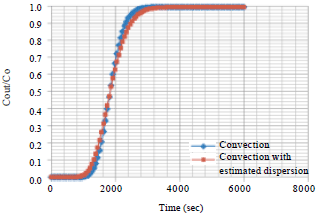
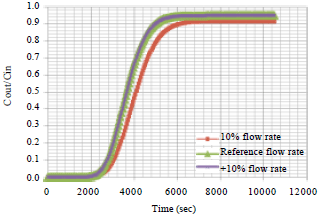
I'm Graduate student and in my study i have to simulate adsorption column for wastewater treatment. and your paper is really very nice paper and very helpful for me.