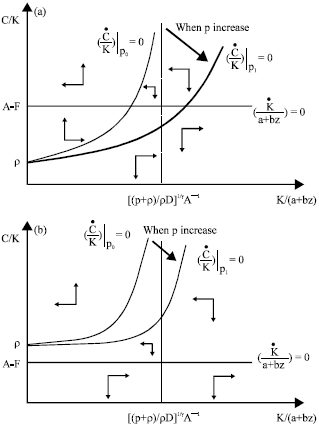
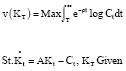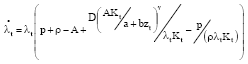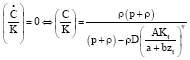Research Article
Research on Nonfarm Economic Optimum Growth Model Considering Environmental Pollution
Tianjin University of Technology Management School, Tianjin, 300384, China
Zhu Yuanyuan
Tianjin University of Technology Management School, Tianjin, 300384, China