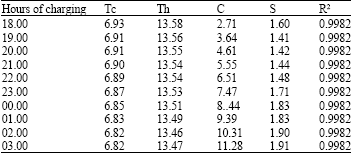
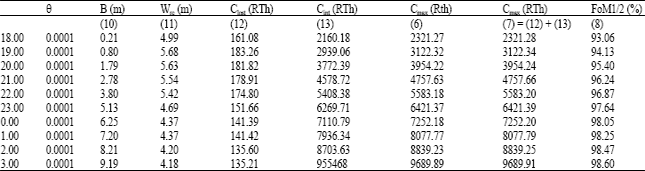
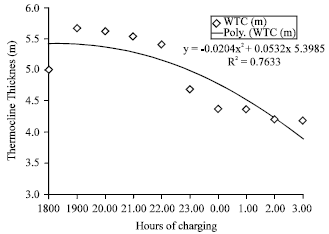
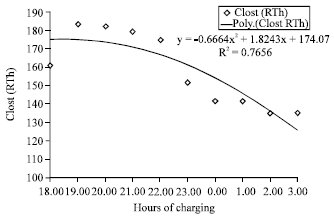
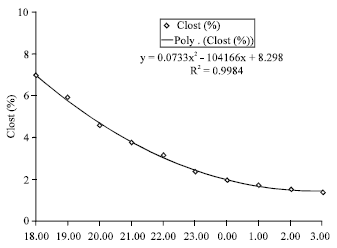
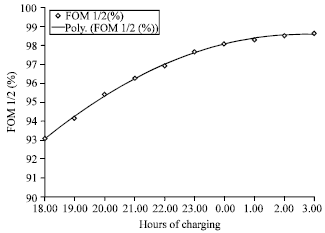
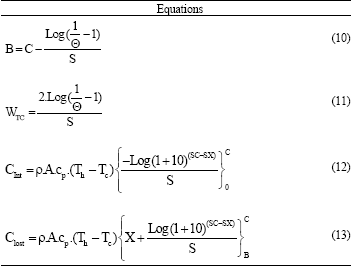Research Article
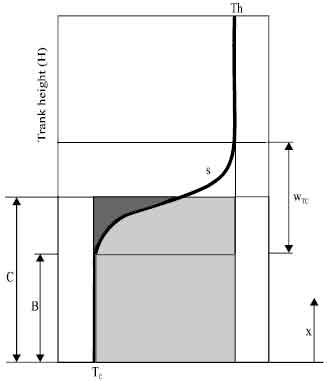
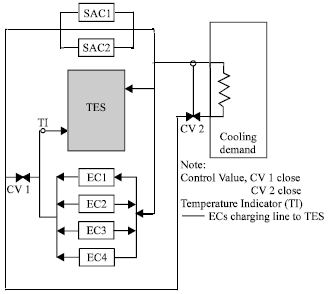
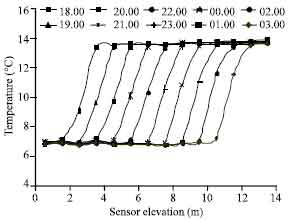
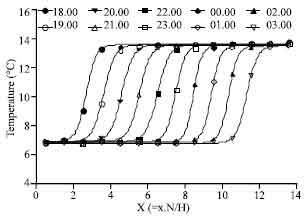
Performance Evaluation of Stratified TES using Sigmoid Dose Response Function
Department of Mechanical Engineering, Universiti Teknologi PETRONAS, Bandar Seri Iskandar, Tronoh 31750, Perak, Malaysia
M. Amin A. Majid
Department of Mechanical Engineering, Universiti Teknologi PETRONAS, Bandar Seri Iskandar, Tronoh 31750, Perak, Malaysia