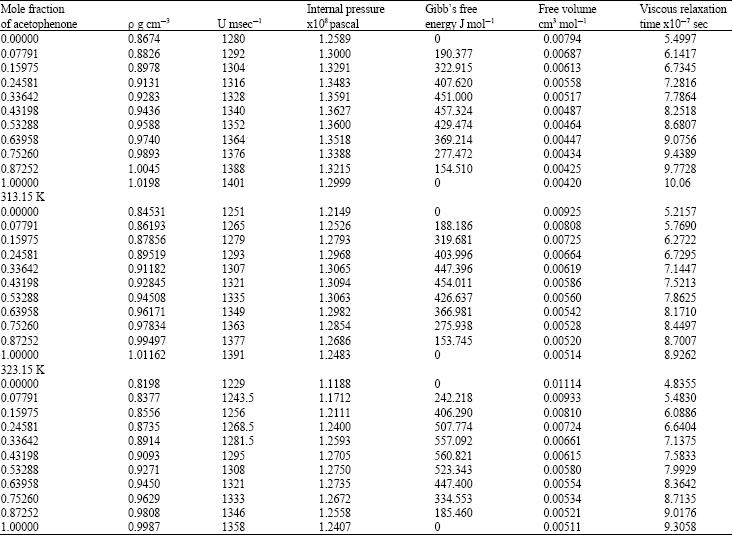
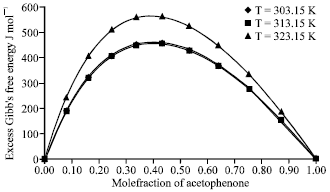
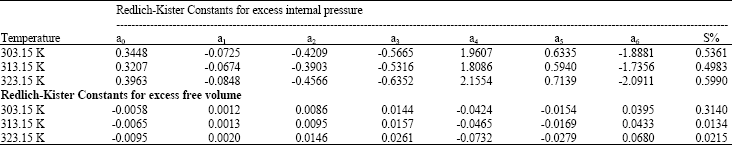
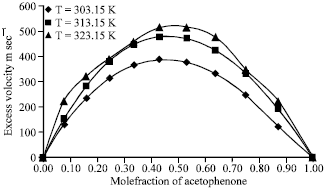
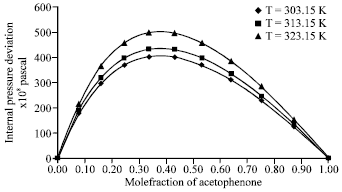
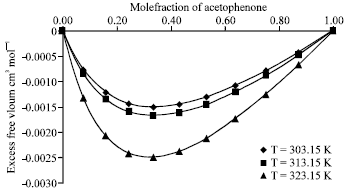
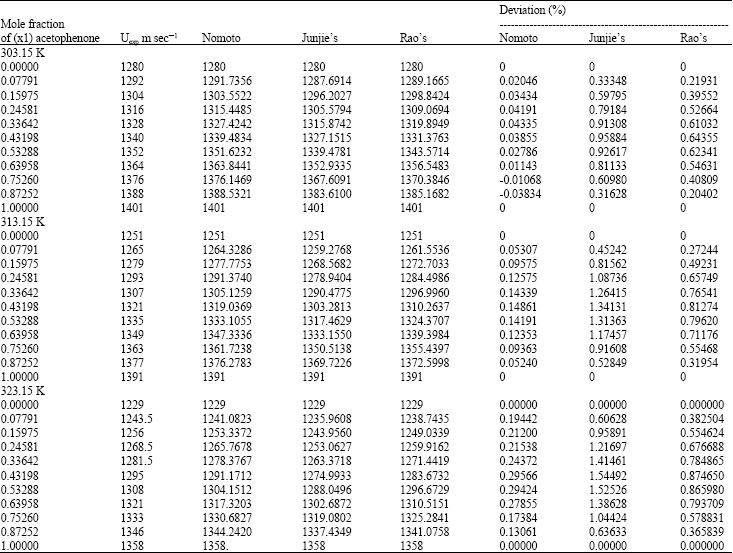
Research Article
Acoustic and Thermodynamic Properties of Binary Liquid Mixtures of Acetophenone and Benzene
Department of Chemical Engineering, St. Peter�s Engineering College, Chennai-54, India
R. Baskaran
Department of Chemical Engineering, St. Joseph�s College of Engineering, Chennai-119, India
T.R. Kubendran
Department of Chemical Engineering, Alagappa College of Technology, Anna University, Chennai-600025, India