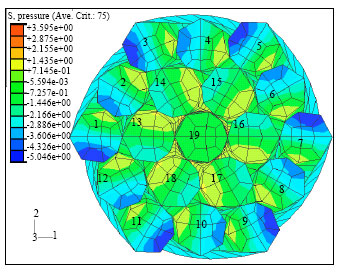
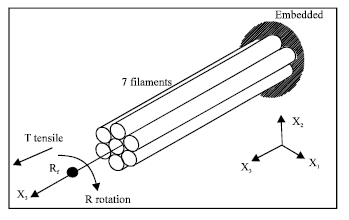
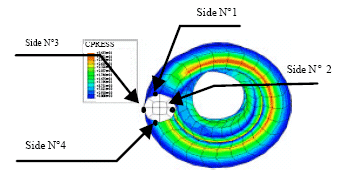
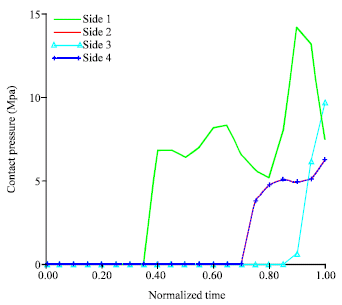
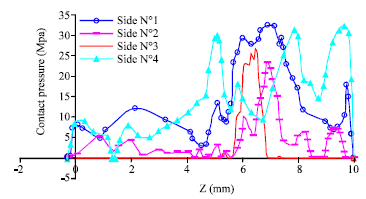
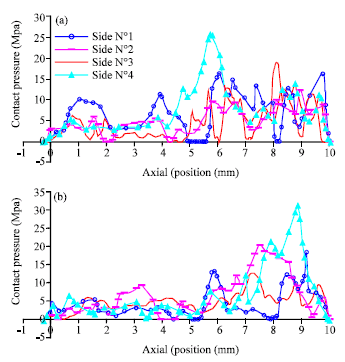
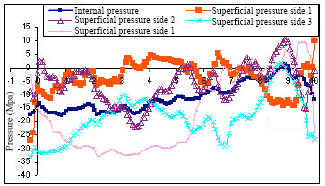
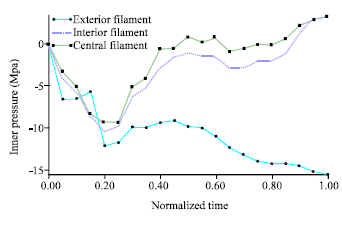
Research Article
Three Dimensional Numerical Study of Contact and Inner Pressures in a Polypropylene Twisted Yarn
Textile Research Unit, ISET of Ksar Hellal, BP. 68, Ksar Hella 5070, Tunisia
S. Msahli
Textile Research Unit, ISET of Ksar Hellal, BP. 68, Ksar Hella 5070, Tunisia
M. Zidi
Laboratory of Mechanical engineering, ENIM, Monastir 5000, Tunisia
F. Sakli
Textile Research Unit, ISET of Ksar Hellal, BP. 68, Ksar Hella 5070, Tunisia