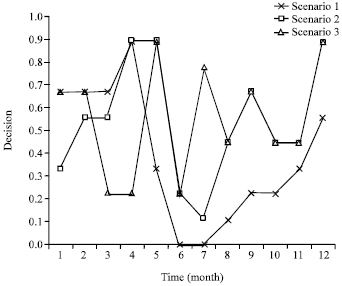
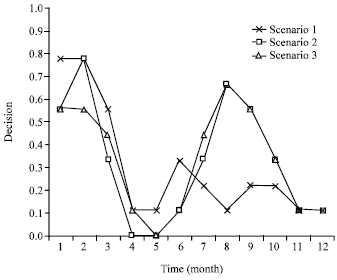
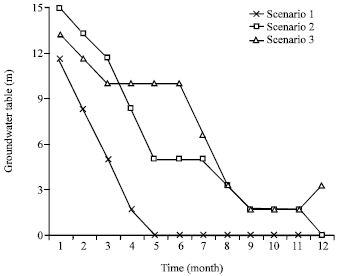
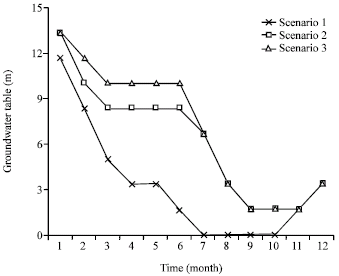
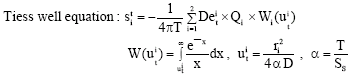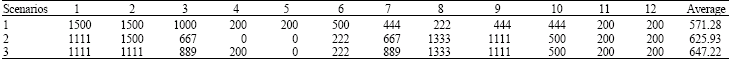Research Article
A Model of Non-Cooperative Dynamic Game to Conflict Resolution Among Common Natural Resource Operators
Faculty of Civil Engineering, Iran University of Science and Technology, Narmak, Tehran, Iran
A. Ghaheri
Faculty of Civil Engineering, Iran University of Science and Technology, Narmak, Tehran, Iran
L. Karp
Faculty of Civil Engineering, Iran University of Science and Technology, Narmak, Tehran, Iran