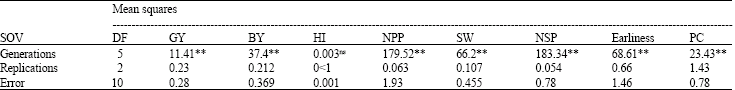
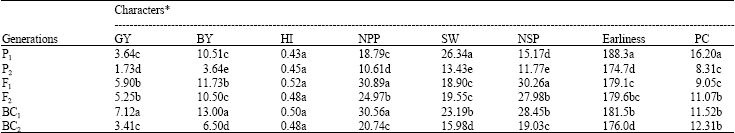
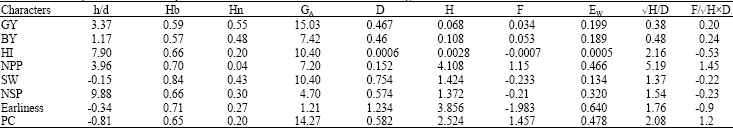
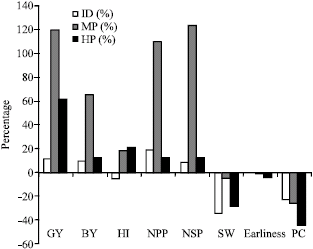
Research Article
Inheritance of Drought Tolerance in Chickpea (Cicer arietinum L.) Using Joint Scaling Test
College of Agriculture, Razi University, Kermanshah, Iran
S.H. Sabaghpour
Dryland Agricultural Research Institute, Sararood, Kermanshah, Iran
N. Khaksar
College of Agriculture, Razi University, Kermanshah, Iran