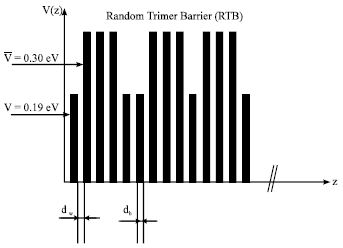
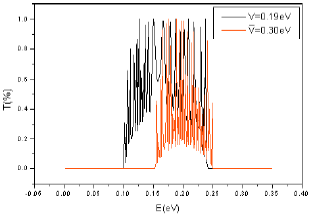
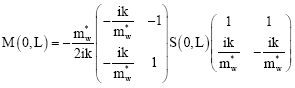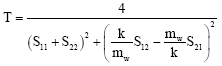Research Article
Electronic States Nature of Trimer Height Barrier Disorder Superlattices
Department of Physique, Faculte des Sciences, Universlte Abdelhamid Ibn Badis B.P. 227 Mostaganem, Algerie
S. Bentata
Department of Physique, Faculte des Sciences, Universlte Abdelhamid Ibn Badis B.P. 227 Mostaganem, Algerie
Z. Aziz
Department of Physique, Faculte des Sciences, Universlte Abdelhamid Ibn Badis B.P. 227 Mostaganem, Algerie