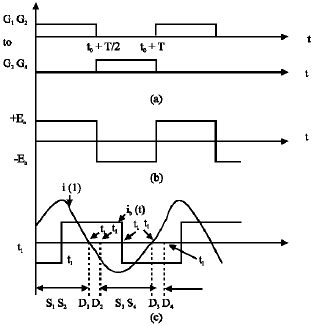
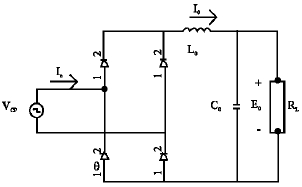
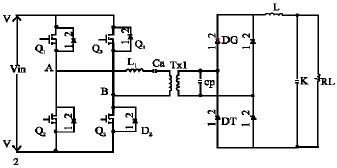
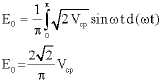Research Article
Analysis Design and Development of Regulated Power Supply-Using Soft Switched Resonant Converters
Department of ECE, AMA Engineering College, Kanchipuram, Tamil Nadu, India
Y. Sukhi
Sathyabama University, Old Mahapalipuram Road, Tamil Nadu, India
Y. Jeyashree
Sathyabama University, Old Mahapalipuram Road, Tamil Nadu, India