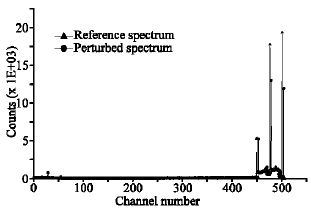
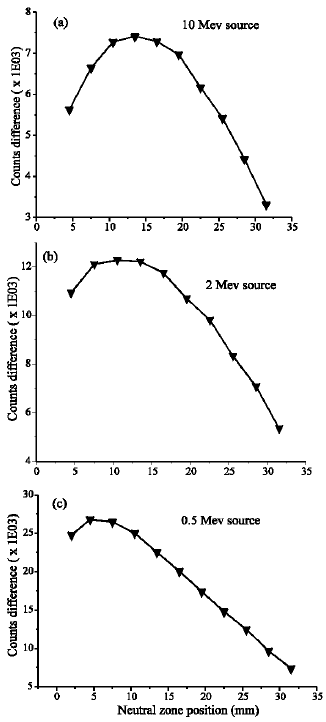
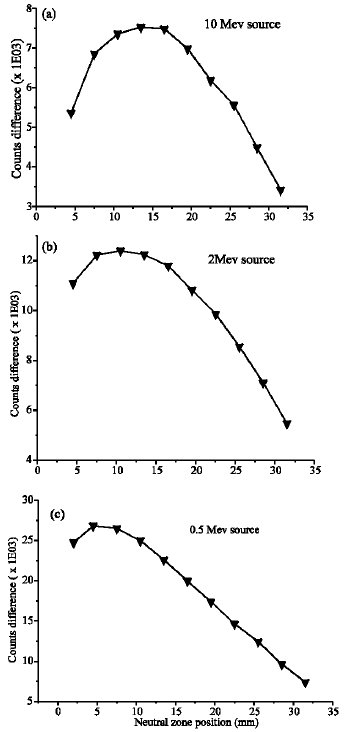
Research Article
Study of Zone Importance for Gamma-Rays in Germanium Detectors by Monte Carlo Simulation
Physics Department, USTO, BP 1505 El-MNouar, Oran, Algeria
A. Oukebdane
Physics Department, USTO, BP 1505 El-MNouar, Oran, Algeria
F.Z. Khiari
Center for Applied Physical Sciences, Research Institute, King Fahd University of Petroleum and Minerals, Dhahran 3 1261, Saudi Arabia