ABSTRACT
The objective of this study is to understand the water induced consolidation process at agriculturally used reclaimed soil. The approach of the study is to reproduce the situation occurring when the soil is subjected to water induced compaction at defined loads typical in the top soil (0 to 20 kPa). The water induced compaction process is characterized by sudden change in the voids or pores of the soil that are loaded at their natural water content and flooded. Compression properties are measured with Oedometer tests using sieved sandy loam, taken from a non weathered Saalian glacial till layer, as the meta-stable test soil. Pre-consolidation load calculated for the dry samples overestimated the simulated overburden pressure, whereas, the wetted samples showed a high congruence. In both cases, the point of greatest curvature estimated in the earlier step of deviation gave values that are more realistic to the loads from which the unloading started. The results indicated that both dry compaction and water-induced compaction induce primarily plastic deformation.
PDF Abstract XML References Citation
How to cite this article
DOI: 10.3923/ijss.2010.26.33
URL: https://scialert.net/abstract/?doi=ijss.2010.26.33
INTRODUCTION
Since, the early 1990’s, in the lower Lusatian lignite-mining district glacial till is used to restore arable land, which has been destroyed due to large-scale lignite mining operations. The glacial till in its natural state before excavation is highly compacted and shows a dense structure, bulk density is in between 1.9 and 2.1 g cm-3 (Narra, 2005). The high bulk density values are regardless, whether, these substrates are used for agriculture or for nature protection purposes.
Investigations on meta-stable arrangements of particles, e.g. by Hartmann et al. (2002) point up that loose arrangements of particles, as it is created by tillage operations, or in this case by mining operation steps like excavation, transport and dumping, are unstable. If wetted, the soil tends to compact rapidly associated with the negative effects on root development of crops (Lesturgez, 2005). This water-induced compaction phenomenon is well known from the loess soil in Lybia (Assallay et al., 1996, 1997) and is further reported for agriculturally used soil in Germany (Horn and Rosteck, 2000). This phenomenon in addition describes the increase in bulk density without additional loads, i.e., only by the weight of the soil itself, when the soil or the particle arrangement is wetted (Narra, 2005). The process behind this phenomenon is that the additional water modifies the stress situation between the soil particles. Associated with this, the equilibrium of the inner forces in the soil becomes unstable and is shifted to a new one (Narra, 2005). The studies concerning the water-induced compaction are primarily focused on the formation of dense layers, so-called fragipans, in agriculturally used soil (Bryant, 1989; Franzmeier et al., 1989; Assallay et al., 1998; Lesturgez, 2005; Narra, 2005).
Studies of water-induced compaction behavior under laboratory conditions are investigated using bench Oedometers with continuously increasing loads. Drained or air-dried soil samples are loaded stepwise to a certain defined stress and are then wetted. When the subsidence in the sample was completed, then the load was further increased. It was found that the disturbed meta-stable soil due to its lower packing state undergoes a greater water-induced compaction compared to undisturbed meta-stable soil previously subjected to both vertical stresses and wetting and drying cycles (Assallay et al., 1997).
Due to the necessity to understand the water-induced compaction process consolidation tests are carried out on natural soil and artificial substrates. The approach of these tests is to reproduce the situation occurring when the soil show water-induced compaction behaviour. The tests consisted of the determination of the dry and saturated consolidation curve of the used soil and the substrate, respectively and then a certain number of combined consolidation tests are carried out. The combined consolidation tests comprised the following steps: (1) loading the dry sample to a certain load, (2) flooding the sample at this load level and (3) the samples are drained to pF1.8 and the saturated samples are loaded stepwise from 0 to 2500 kPa to the maximum stress value.
The goal of this study is to prove the approach to predict the internal stress after water-induced compaction (hydro-collapse) on the basis of consolidation tests with disturbed glacial till samples, which are formerly subjected to defined stress values representing defined depths of the soil. Further, consolidation tests with undisturbed wet and disturbed dry samples shall prove the changes in the internal stress due to mining operations.
MATERIALS AND METHODS
The Saalian glacial till is from the lower Lusatian lignite-mining district located about 100 km Southeast of Berlin, Germany. The test material was collected in October 2006 from the pre-cut section at the Northernmost lignite opencast mining pit Jänschwalde (51°49' N, 14°34' E) from depths of 3 to 10 m at an interval of 1 m. The sediment layer stretches exclusively over the mining area having a mean thickness of approximately 20 m. The study was carried out from Oct 2006 to May 2007. The glacial till is of sandy texture, coherent structure (69.7% sand, 25.8% silt, 4.5% clay) and slightly calcareous (4.3% of CaCO3). The glacial till is classified as loamy sand (USDA soil texture classification).
Void ratio (e) values are obtained from bulk density (ρsoil) and particle density (ρparticle) with the help of Eq. 1. The measured particle density of 2.65 mg m-3 (Stock, 2005) was used in the calculation.
| (1) |
The carbonate content was determined using a Scheibler-Dietrich apparatus. The Scheibler method involves the determination of the carbonate content of a soil based on a volumetric method. The carbonates contained in the sample are converted into CO2 by adding hydrochloric acid to the sample. As a result of the pressure of the CO2 released, the water in a burette that is de-aerated rises. The difference in level measured is an indication for the released quantity of CO2, from which the carbonate content can be calculated. The carbonate content is expressed as equivalent calcium carbonate content. The equation for the calculation of amount of calcium carbonate and the conversion tables are taken from Barsch and Billwitz (1990).
The impact of disturbance on the stability (pre-consolidation load) of the glacial till is evaluated using three sub-steps. The first step comprised of determination of the stress-strain behaviour in undisturbed soil samples reflecting the undisturbed conditions. In the second step, evaluation of the stress-strain behaviour on dry soil samples was carried out, which reflects the situation during summer when the glacial till dries due to reduction in ground water level. With the specific saturation of dry pre-loaded soil samples, in the third step, the effect of saturation and re-compaction by rain was simulated. The goal of this study was to model the effective stress in the re-wetted soil based on the stress-strain behavior of the soil. It shall be shown that the stress obtained corresponds to the overburden load. The above described approach is exemplarily carried out for undisturbed sediment samples and for disturbed dry sediment samples from two depths (4 and 8 m). The two different depths (4 and 8 m) chosen for evaluating water induced compaction are based on the content of carbonates present. The carbonate content divided the profile clearly into 2 different layers. First layer (up to 4 m) is free of carbonate having a mean value between 0.07 and 0.1%. The second deeper layer (from 8 m) characterizes the carbonate content to be about 5.3 to 5.4%. The alteration of carbonate content was at 6 m depth.
The pre-consolidation load is estimated for both the dry and wetted soil using the Casagrande (1936) and Silva (1970) methods, whereas the point of maximum curvature and virgin compression line are mathematically determined using the zero-point of 2nd derivation and 1st zero-point of the 3rd derivation of Eq. 2 as proposed by Baumgartl and Kock (2004). Equation 2 describes the hydraulic model of van Genuchten (1980) in the following modified way:
| (2) |
where, emax and emin being the maximum and minimum void ratio values, when the applied pressure α→4 and α, n and m are the empirical parameters. The parameters emin, α and n are free fitted, whereas m is calculated as displayed in Eq. 2.
The undisturbed soil samples are saturated and drained at a water tension of 63.1 hPa (pF 1.8) on suction plates. The samples are then placed in Oedometer and the different stresses are applied. The resultant height difference of the samples at each stress level has been recorded. In the case of disturbed dry samples, the samples are subjected to different stresses in the Oedometer and for the disturbed saturated samples, the samples are placed on the suction plates with different applied pre-loads (0, 5, 10 and 20 kPa) at a water tension of 63.1 hPa. Then, the samples are placed in Oedometer and are subjected to different stresses.
RESULTS
Water Retention Characteristics
The profile of the pre-cut section can be dissected into two sections from the curves water content vs. water tension: (1) curve obtained from 4 m depth and (2) curve obtained from 8 m depth. It can be seen that the samples from 4 m do not have coarse pores as the drainage of the samples start from the point above field capacity, whereas the samples from 8 m have coarser pores as the drainage starts below the field capacity value (Fig. 1a, b).
At 4 m depth the curve showed a steeper gradient and the difference in the water content values of the saturated and the point of permanent wilting point is about 0.15 cm3 cm-3. At 8 m depth the samples displayed maximum saturation water content and the increase in drainage to be gradual. On the basis of water retention curves, the saturated water content (1 hPa or pF0), field capacity (63.1 hPa or pF1.8) and permanent wilting point (15849 hPa or pF4.2) are calculated and are given in Table 1.
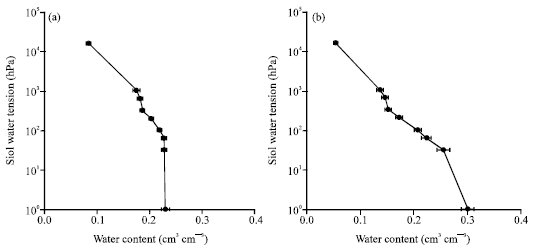 | |
| Fig. 1: | Water retention curves (pF-Curve) at (a) 4 and (b) 8 m depths of the pre-cut section in lignite mining pit Jänschwalde (5 replicates from each depth) |
| Table 1: | Values of saturated water content, field capacity, permanent wilting point at different depths |
 | |
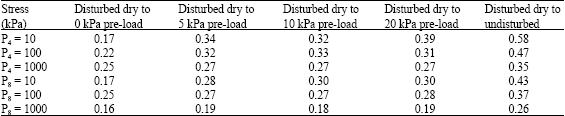
| Table 2: | Magnitude of water-induced compaction based on the differences in void ratios at 10, 100 and 1000 kPa |
 | |
| P4 = 4 m depth, P8 = 8 m depth | |
The results showed that the water content measured at 4 and 8 m depths was around 20% volume and 30% volume higher compared to water content measured at permanent wilting point. The huge amount of water content can cause in sudden collapses noted as water-induced compaction. This collapse would be plastic and is not reversible. This high water content also resulted in swelling and shrinkage of the profile. Swelling of the samples has been observed when the samples are completely saturated on the suction plate, especially from the 8 m depth samples. Shrinkage has been observed in the samples from 4 m depth when the samples are completely drained at pF4.2.
Water-Induced Compaction
The water-induced compaction of the meta-stable soil is evaluated at three different stress levels (10, 100 and 1000 kPa). The dry test soil displays a considerable capacity to restrict compaction (Fig. 2). The load capacity of soil decreases abruptly with wetting and the soil collapses to a certain value of void ratio described by the compression curves of the wet soil. As a result, the water-induced compaction rates were higher in between 10 and 100 kPa with a maximum at 100 kPa (Table 2). The relation between the added quantity of water and the void-ratio changes during the water-induced compaction for stress levels of 10, 100 and 1000 kPa can be observed in Fig. 2a and b.
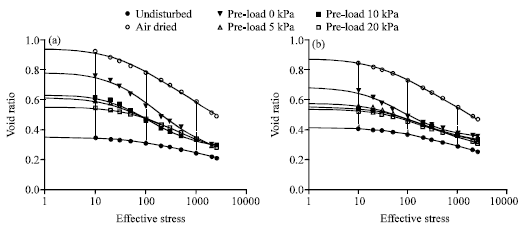 | |
| Fig. 2: | Pre-consolidation curves plotted for undisturbed, disturbed dry and disturbed wet samples from (a) 4 and (b) 8 m depths |
| Table 3: | Maximum and minimum void ratio values obtained from 4 and 8 m depths for undisturbed, disturbed dry and disturbed saturated samples with pre-loads |
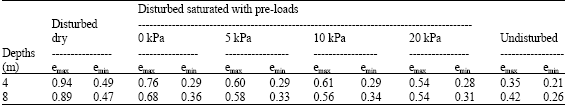 | |
From Fig. 2 it becomes evident, that the water-induced compaction already commenced when the soil is still in the unsaturated state. At this point, about 20% of the pore space is filled with water. Disturbed saturated samples from both 4 and 8 m depths showed a decrease in the initial void ratio value with the increase in the preloaded stress (0, 5, 10, 20 kPa). The final void ratio values of all the disturbed wet curves were almost equal. The observations showed that with the increase in the pre-loads, the curves get closer to each other and assume a flatter shape similar to the shape of the curve obtained from undisturbed samples.
The difference between the void ratio values of the disturbed dry and the disturbed saturated curves gives the magnitude of water-induced compaction. Initial void ratio and final void ratio values are given in Table 3. The difference between the initial void ratio and the final void ratio values were less in the case of 8 m samples compared to that of 4 m samples. This difference in void ratio endangers the intensity of water-induced collapse. Intensity of collapse was high with higher void ratio difference. The disturbed saturated curve pre-loaded with 20 kPa stress has a similar shape as that of the undisturbed samples. This indicates that the disturbed saturated samples are taking the shape of the undisturbed samples with increasing pre-loads. So to find out the value at which the initial void ratio value of the disturbed wet curve will be same as that of the initial void ratio value of the undisturbed curve. The stress required was 900 kPa for 4 m depth samples and 600 kPa for 8 m depth samples. The undisturbed samples are taken from the pre-cut section which is with out any external loads acting over it, but still we saw that the soil particles are ideally packed to have such high stresses, this can be due to the oscillation of the glacial till under ice glaciation.
| Table 4: | Pre-consolidation loads calculated for undisturbed, disturbed dry and disturbed saturated samples with pre-loads from 4 and 8 m depths |
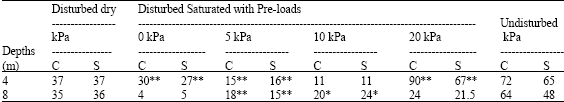 | |
| Overestimation: *Two fold higher, **Three fold higher. C: Casagrande (1936), S: Silva (1970) | |
Pre-Consolidation Load Before and after Wetting
The pre-consolidation load before and after water-induced compaction is mathematically estimated from the recompression data using Eq. 2. For the dry samples, all modeled curves fit the recompression data, although, the curves underestimate the measured void ratio values in the range of the transition between the recompression and virgin compression curve in all cases (Fig. 2).
Pre-consolidation load was calculated using the inflection point and the point of maximum curvature, reproducing overburden-pressure values distinctly higher than the stress from which the soil has been unloaded. The point of maximum curvature also gave values that are distinctly different to the applied stress levels.
For wetted samples, all modeled curves fit the recompression data with high accuracy, although for Pw = 10 kPa, an underestimation in the range of the transition between the recompression and virgin compression curve was observed. Table 4 gives the values of the pre-consolidation loads measured from 4 and 8 m depths. The pre-consolidation loads are overestimated in the case of 4 m depth samples; whereas, the samples from 8 m depth are in close correlation to the simulated overburden pressures (pre-loads). However, the point of maximum curvature gave values that are more realistic to the simulated overburden pressures.
DISCUSSION
The results of the experiments on water-induced compaction displayed in Fig. 2 show that the collapse rates for the investigated glacial till can be easily estimated from the dry and wet-compaction curves. This water-induced compaction is useful in determining the collapse potential of naturally and artificially compacted soils. The range of stresses that usually occur when the glacial till is subjected to cover spoil heaps are in between 10 and 100 kPa, which is equivalent to a cover-layer thickness of 2 to 10 m.
Under laboratory conditions, the maximum void-ratio changes and therewith the highest potential of the meta-stable soil to collapse has been detected in the range of 10 to 1000 kPa. The congruence of the stresses induced by the overburden pressure after deposition of the glacial till and the stress range of maximum collapse rates show that the glacial till is highly sensitive to wetting under the naturally occurring field conditions. This phenomenon of collapse due to the induced water in the glacial till has also been observed by Stock (2005). The sensitivity of the glacial till material to wetting is due to the treatment of soil in which it is transformed into a meta-stable soil structure after sieving. It should be noted that this treatment is comparable to the field conditions in which excavation, transport and deposition activities disrupt the soil structure (Narra, 2005). The assumptions with the disruption of soil structure are consistent with model experiments on loess investigated by Assallay et al. (1997) and for sandy soil investigated by Lesturgez (2005). Assallay et al. (1997) and Lesturgez (2005) demonstrated that the disturbances in the natural soil will be decreasing with wetting and is related to their load bearing capacity, while the undisturbed loess soil have their maximum collapse at stresses >1000 kPa as also observed by Assallay et al. (1996).
From Fig. 2, the initiation of water-induced compaction is seen to occur before the soil is saturated. This is interesting since, it shows that the risk of water-induced collapse begins at a very early state of saturation or in other words, the threshold for critical water content appears to be approximately 20% or more water-filled pore space.
However, this is only theoretical since, it is not clearly known, whether, the water is homogeneously distributed throughout the soil or only at the beginning of the collapse event, i.e., only in the top few millimeters of the soil (Stock, 2005; Narra, 2005). Further, it became clear that the collapse occasionally continued to occur after the soil has been saturated, especially at 100 kPa, where the soil showed the highest rate of collapse (Table 2). The collapse continues over a longer period than that of the actual saturation, since the collapse rate is slower than the saturation process.
Baumgartl and Kock (2004) have shown that the pre-consolidation load can be estimated purely mathematically using the sigmoidal fit function described by Van Genuchten (1980) to derive the parameters needed for the Casagrande (1936) and Silva (1970) methods. The sigmoidal Van Genuchten (1980) model presumes a continuous run of the data, which needs to be fitted. The Casagrande (1936) method is highly subjective and varies from person to person in finding out the point of maximum curvature. This problem is solved by the Silva (1970) method and the estimated pre-consolidation values are not subjected to interpretation. Therefore, the value obtained will not be prone to errors (Narra, 2009).
CONCLUSION
The water-induced compaction rates of the metastable test soil derived using the dry and wet compression curves are found to be highest between 10 and 1000 kPa. The simulated water-induced compaction with both disturbed and disturbed saturated conditions indicate a irreversible (plastic) deformation of the meta-stable soil. The pre-consolidation load provides a useful prediction of the overburden pressure after deposition under field conditions. The derived overburden-pressure (pre-loads) values are more realistic after wetting for 8 m depth samples compared to 4 m depth samples.
REFERENCES
- Assallay, A.M., C.D.F. Rogers and I.J. Smalley, 1996. Engineering properties of loess in Libya. J. Arid Environ., 32: 373-386.
CrossRef - Assallay, A.M., C.D.F. Rogers and I.J. Smalley, 1997. Formation and collapse of metastable particle packings and open structures in loess deposits. Eng. Geol., 48: 101-115.
CrossRef - Assallay, A.M., I. Jefferson, C.D.F. Rogers and I.J. Smalley, 1998. Fragipan formation in loess soils: Development of the Bryant hydroconsolidation hypothesis. Geoderma, 83: 1-16.
CrossRef - Barsch, H. and K. Billwitz, 1990. Physisch-geographische Arbeitsmethoden. Hermann Haack, Germany, ISBN: 3730108573, pp: 256.
Direct Link - Baumgartl, Th. and B. Kock, 2004. Modeling volume change and mechanical properties with hydraulic models. Soil Sci. Soc. Am. J., 68: 57-65.
Direct Link - Narra, S., 2009. Influence of compaction curve modeling on void ratio and pre-consolidation stress. Int. J. Soil Sci., 4: 57-66.
CrossRefDirect Link - Van Genuchten, M.T., 1980. A closed-form equation for predicting the hydraulic conductivity of unsaturated soils. Soil Sci. Soc. Am. J., 44: 892-898.
CrossRefDirect Link








