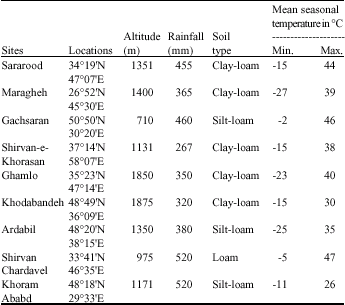
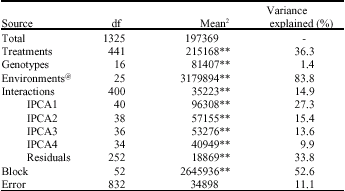
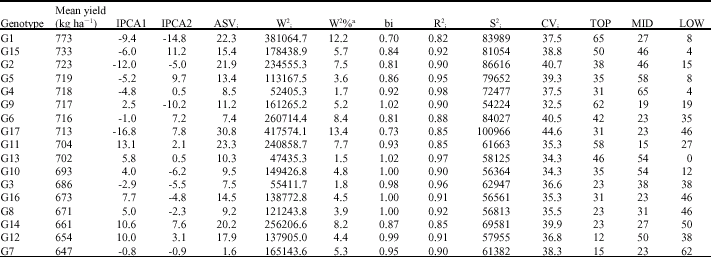
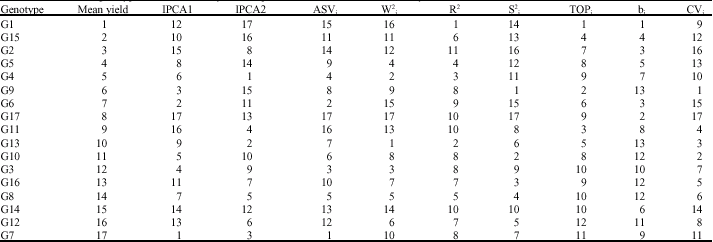
Research Article
Use of Stability Parameters for Comparing Safflower Genotypes in Multi-Environment Trials
Dryland Agricultural Research Institute, P.O. Box 67145-1164, Kermanshah, Iran
Reza Mohammadi
Dryland Agricultural Research Institute, P.O. Box 67145-1164, Kermanshah, Iran