An Analytic Proof of Bugeaud-Mignotte Theorem

The Bugeaud-Mignotte theorem concerns the Diophantine equation , when X = 10. It includes the fact that any integer greater than 2 and with all digits equal to 1 in base ten cannot be a pure power. It means that does not exist Y an integer strictly greater than 1 and q an integer strictly greater than 1 for which Y^q is a number with all digits equal to 1 in base ten.

INTRODUCTION

11, 111, 1111 in base ten are not pure powers, we can easily verify it, but is it true for a number of n digits equal 1 in base ten, for all n. This study is an answer to this question. A number with n digits equal to 1 (111…1) in base ten is equal to a number with n digits equal to 9 (999…9) in base ten divided by 9, which means , the theorem stipulates that , . This research proposes an original and analytic proof of this theorem called Bugeaud and Mignotte theorem (Bugeaud and Mignotte, 1999).

THE PROOF

Let Bugeaud-Mignotte equation:

Then with j2 = -1

10n-9Yq = 1 = 10n+j(9jYq)

We pose:

10n = x, 9jYq = y, u = 1, z = 9jYq10n

Lemma 1

(1)

(2)

Proof of Lemma 1

And:

We pose:

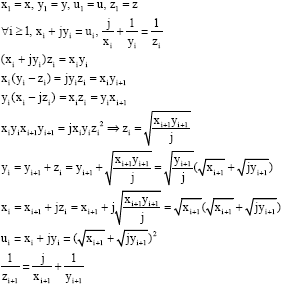until infinty.

Lemma 2

The expression of the sequences is

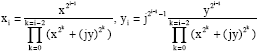Proof of Lemma 2

For I = 2, , it is verified. We suppose the expressions true for I

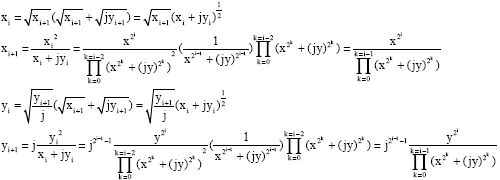But:

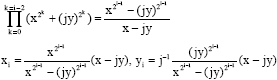Lemma 3

x_i-jy_i = x-jy

Lemma 4

The lemmas 1, 2, 3 imply that jy-x = 0

Proof of Lemma 4

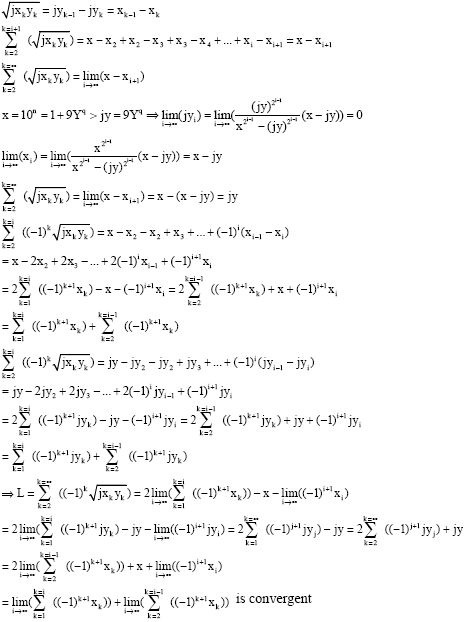We pose:

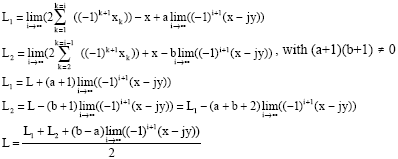We pose:

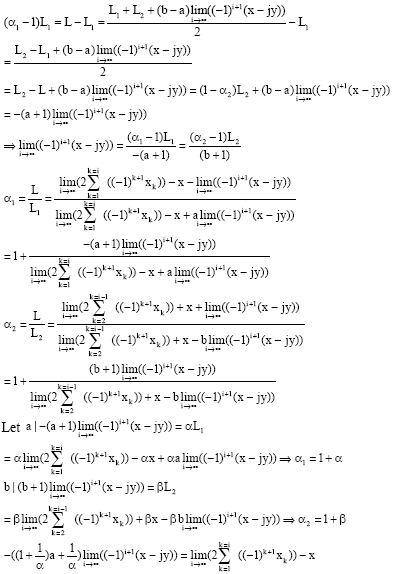And:

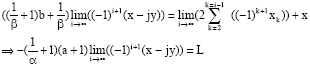And:

If we make the hypothesis that

And:

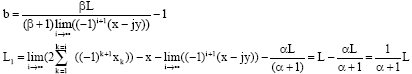But:

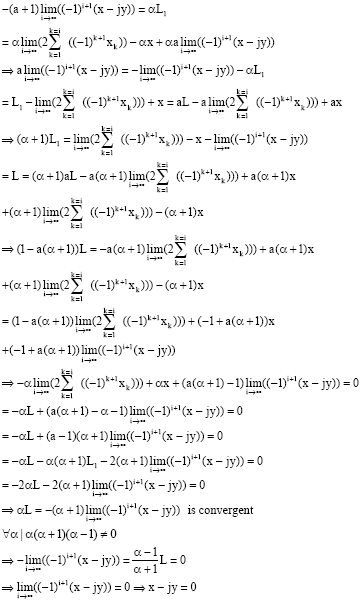The hypothesis is false and

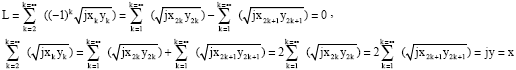In all cases

is convergent, then the general term of the series tends to zero

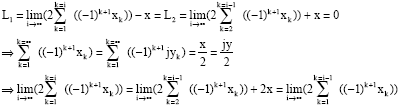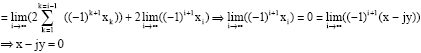And it is impossible: the existence of Y and q is impossible!

CONCLUSION

Bugeaud-Mignotte equation has effectively no solution, and an analytic proof exists. The generalization is is it possible? It seems that there are only three solutions: